What are the products for the alpha decay of ""_64^150Gd15064Gd?
1 Answer
Here's what I got.
Explanation:
Alpha decay is said to take place when a radioactive nuclide decays by emission of an
 )
)
So right from the start, you know that one of the products will be an
""_(color(white)(x)64)^150"Gd" -> color(blue)(""_x^y?) + ""_2^4"He"150x64Gd→yx?+42He
In order to determine the identity of that
Mass number, which represents the number of protons and neutrons located in the nucleus, is written in the upper-left of the chemical symbol.
The atomic number, which tells you the number of protons located in the nucleus, is written in the lower-left of the chemical element.
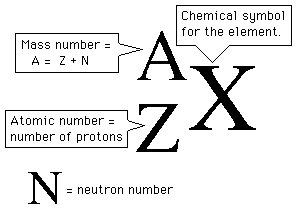
So, looking at your nuclear equation, you can say that
150 = y + 4 ->150=y+4→ conservation of the mass number**
and
64 = x + 2 ->64=x+2→ conservation of charge**
This will give you
{(y = 150 - 4 = 146), (x = 64 - 2 = 62) :}
A quick look in the periodic table will reveal that samarium,
""_(color(white)(a)64)^150"Gd" -> ""_62^146"Sm" + ""_2^4"He"

