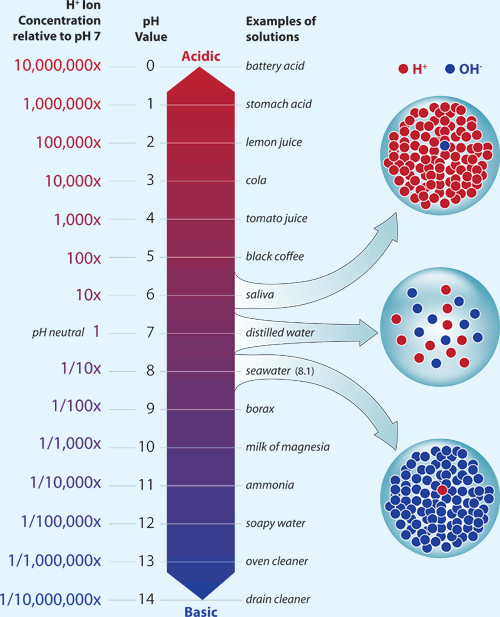
What does it mean if a solution has a pH of 6.0?
1 Answer
That it contains
Explanation:
As you know, the
More specifically, to find the
#"pH" = - log(["H"_3"O"^(+)])#
You can rewrite this equation as
#["H"_3"O"^(+)] = 10^(-"pH")#
Now, pure water at room temperature has
#"pH" = 7#
This implies that the concentration of hydronium cations in pure water at room temperature is equal to
#["H"_3"O"^(+)] = 10^(-7)color(white)(.)"M"#
#["H"_3"O"^(+)] = 1 * 10^(-7)color(white)(.)"M"#
In order for the
So for
#["H"_3"O"^(+)] = 10^(-6.0)color(white)(.)"M"#
#["H"_3"O"^(+)] = 1 * 10^(-6)color(white)(.)"M"#
This corresponds to an increase by an order of magnitude in the concentration of hydronium cations, since
#(["H"_ 3"O"^(+)]_ "pH = 6.0")/(["H"_ 3"O"^(+)]_ "pH = 7") = (1 * 10^(-6)color(red)(cancel(color(black)("M"))))/(1 * 10^(-7)color(red)(cancel(color(black)("M")))) = 10#
You can thus say a solution that has a
This, of course, implies that you are dealing with a solution that is slightly acidic, since that is what