Question

What is the area of this regular hexagon?

Answer 1

`75 cm^2`

Area =`1/2 * 48 *5 cm^2 = 120cm^2`

Explanation:

We will Use the area of the hexagon formula

`Area = 1/2` x perimeter x apothem
Now what is is an apothem;
The apothem (sometimes abbreviated as apo) of a regular polygon is a line segment from the center to the midpoint of one of its sides. Equivalently, it is the line drawn from the center of the polygon that is perpendicular to one of its sides.

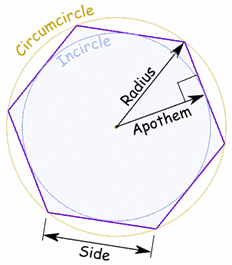
Apothem`= 5 cm` Side `= 8 cm`

Now for a regular polygon of n sides the perimeter is `= n*s`

`= 6*8 = 48`

Finally lets plug in ;

Area =`1/2 * 48 *5 cm^2 = 120cm^2`

Additionally there are multiple ways to find area of a hexagon

1)Use formula ; If you know a side of a regular hexagon you can use this;

2) Using the apothem method;

For more on this visit;

http://www.dummies.com/how-to/content/how-to-calculate-the-area-of-a-regular-hexagon.html

Answer 2

The area is approximately `86.6 "cm"^2`.

Explanation:

As this hexagon is regular, you can divide it into `6` triangles.

Please note that all those triangles are isosceles. All angles of the hexagon are `120°`.

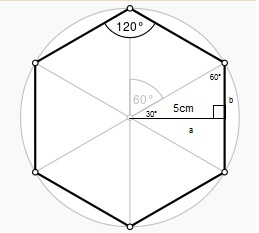
As you see in the picture, each of those 6 "big" triangles can be divided into two small triangles with the angles `30°`, `60°` and `90°`, and we know the length of one of the sides: `a = 5 cm`.

To compute the area of the small right angle triangle, you need just the length of `b`.

This you can do with `tan`:

`tan (30°) = b/a`

`b = 5 "cm" * tan(30°) = 2.88675134595... "cm"`

This means that the area of the small right angle triangle is

`A_t = (b * a)/2 = (5 "cm" * 5 "cm" tan(30°))/2 = 25/2 tan(30°) "cm"^2 = 7.21687836487... "cm"^2`

There are `12` equal triangles, so the area of the whole hexagon is

`A = 12 * A_t = 6 * 25 tan(30°) "cm"^2 = 86.6025403784... "cm"^2`

Hope that this helped!