Question

What is the orthocenter of a triangle with corners at `(3 ,1 )`, `(4 ,5 )`, and (2 ,2 )#?

Answer 1

Orthocenter of the triangle ABC is `color(green)(H (14/5, 9/5)`

Explanation:

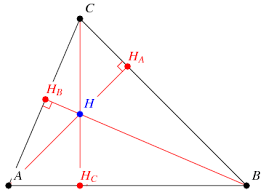
The steps to find the orthocenter are:
1. Find the equations of 2 segments of the triangle (for our example we will find the equations for AB, and BC)

1. Once you have the equations from step #1, you can find the slope of the corresponding perpendicular lines.

2. You will use the slopes you have found from step #2, and the corresponding opposite vertex to find the equations of the 2 lines.

3. Once you have the equation of the 2 lines from step #3, you can solve the corresponding x and y, which is the coordinates of the orthocenter.

Given (A(3,1), B(4,5), C(2,2)

Slope of AB `m_c = (y_B - y_A) / (x_B - x_A) = (5-1) / (4-3) = 4`

Slope of `AH_C` `m_(CH_C) = -1 / m_(AB) = -1/4`

Similarly, slope of BC `m_a = (2-4) / (2-5) = 2/3`

Slope of `(AH_A)` `m_(AH_A) = (-1 / (2/3) = -3/2`

Equation of `CH_C`

`y - 2 = -(1/4) (x - 2)`

`4y + x = 10` eqn (1)

Equation of `AH_A`

`y - 1 =- (3/2) (x - 3)`

`2y + 3x = 12` Eqn (1)

Solving equations (1), (2), we get the coordinates of Orthocenter H.

`color(green)(H (14/5, 9/5)`