What is the osmotic pressure of a 0.050 M solution of #AlCl_3# in water that is at 0.00°C?
Consider #AlCl_3# to be a strong electrolyte.
Consider
1 Answer
Explanation:
Osmotic pressure is all about the number of particles of solute present in solution.
As you know, a solution's osmotic pressure represents the minimum pressure that must be applied in order to prevent water from flowing inward through a semipermeable membrane.
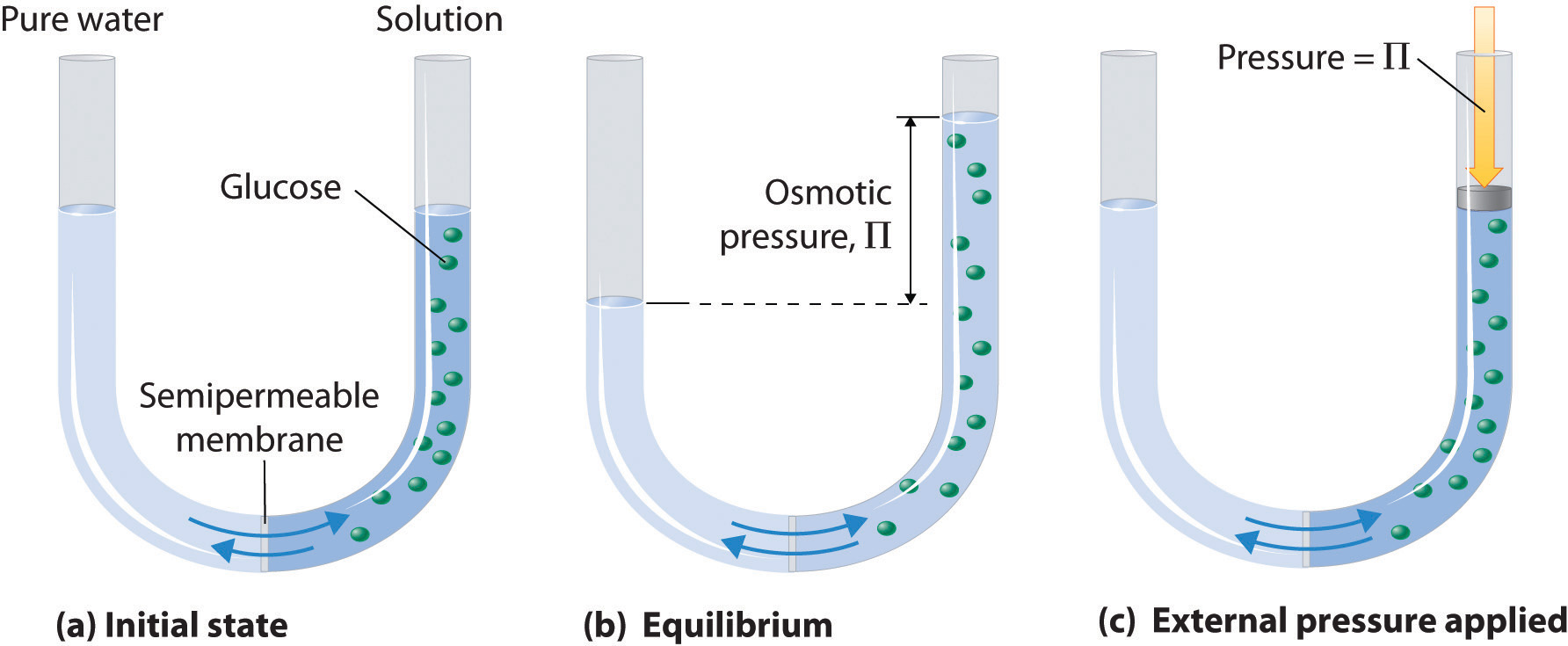
Osmotic pressure is directly related to osmosis, which represents the movement of water through a semipermeable membrane from a region of lower solute concentrations to a region of higher solute concentration.
Simply put, the higher the concentration of particles of solute in a solution, the greater the intake of water through the semipermeable membrane, and thus the higher the osmotic pressure.
Osmotic pressure,
#color(blue)(|bar(ul(color(white)(a/a)Pi = i * c * RTcolor(white)(a/a)|))#
Here
Now, you're dealing with a
Aluminium chloride dissociates completely in aqueous solution to produce aluminium cations and chloride anions
#"AlCl"_ (3(aq)) -> "Al"_ ((aq))^(3+) + 3"Cl"_ ((aq))^(-)#
Notice that every mole of aluminium chloride that dissociates produces
The van't Hoff factor essentially tells you how many moles of particles of solute you get per mole of solute dissolved in solution. In this case,
#i = 4#
Plug in your values into the above equation and solve for
#Pi = 4 * 0.050 color(red)(cancel(color(black)("mol")))/color(red)(cancel(color(black)("L"))) * 0.0821("atm" * color(red)(cancel(color(black)("L"))))/(color(red)(cancel(color(black)("mol"))) * color(red)(cancel(color(black)("K")))) * (273.15 + 0.00)color(red)(cancel(color(black)("K")))#
#Pi = color(green)(|bar(ul(color(white)(a/a)color(black)("4.5 atm")color(white)(a/a)|)))#
The answer is rounded to two sig figs.

