Question

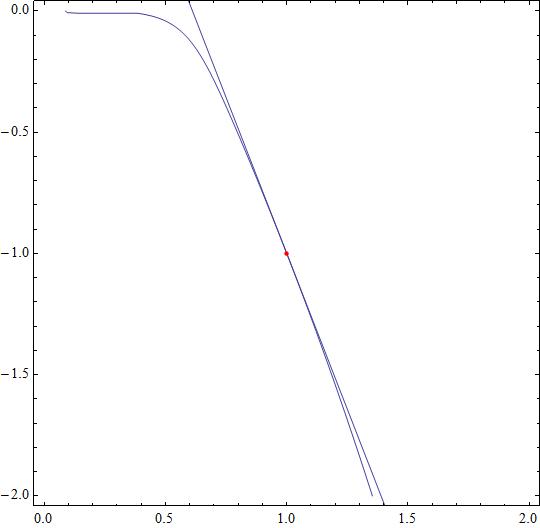
What is the slope of the tangent line of `1/(x^2-y)-y/x+x^3/y = C`, where C is an arbitrary constant, at `(1,-1)`?

Answer 1

The tangent line is `y = -1 - 18/7 (x-1)`

Explanation:

Given `f(x,y,C)=0` if `p_0 = {1,-1} in f(x,y,C)=0-> f(1,-1,C)=0->C=1/2`
Now the tangent space to `f(x,y,1/2)=0` is given by

#(dy)/(dx)=-(f_x)/(f_y) =y (1/x + (2 x^7 - 2 x^2 y - 4 x^5 y + y^2 + 2 x^3 y^2)/( x^8 - 2 x^6 y + x (2 x^3-1) y^2 - 2 x^2 y^3 + y^4))#

evaluated at `p_0` gives `((dy)/(dx))_0=-18/7` and the tangent line is given by

`y = -1 - 18/7 (x-1)`