Question

What is the volume of the solid produced by revolving `f(x)=abssinx-abscosx, x in [pi/8,pi/3]`around the x-axis?

Answer 1

`pi/48( 10 pi-12(1+sqrt 2))=0.16005` cubic units, nearly.

Explanation:

I supplied the missing the factor 1.2 in the integral of sin 2x now. I

am sorry for missing it, in my earlier version..

`[pi/8, pi/3] in (0, pi/2)`.

Both sin x and cos x are > 0 in (0, `pi/2`). So,

`|sin x|-|cos x|=sin x - cos x, x in [pi/8, pi/3]`.

Now, the volume of solid of revolution is

`pi int (sinx -cos x)^2 d x`, between the limits `pi/8 and pi/3`

`=pi int (sin^2x+cos^2x-2 sinx cos x) d x`, between the limits

`=pi int (1-sin 2x) d x`, between the limits

`=pi [x+1/2cos 2x]`, between the limits

`=pi((pi/3-pi/8)+1/2(cos (2/3pi)-cos(pi/4))`

`=pi (5/24pi+1/2(-1/2-1/sqrt 2))`

`=pi/48(10pi-12(1+sqrt2))` cubic units.

Answer 2

Interesting fact.

Explanation:

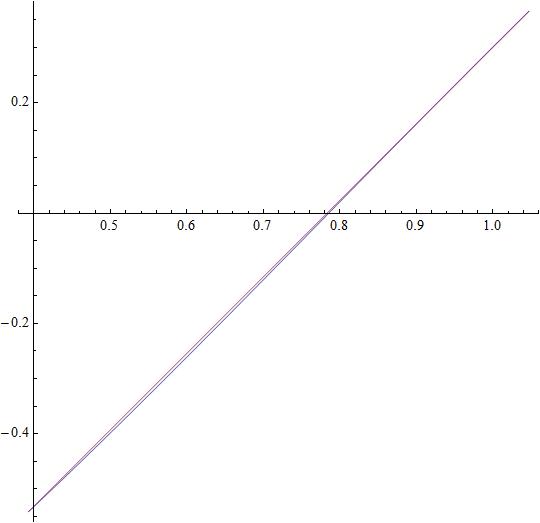
Comparing `f(x) = sinx-cosx` for `x in [pi/8,pi/3]` with
`y = y_1 + (y_2-y_1)/(x_2-x_1)(x-x_1)` in which

`x_1 = pi/8, y_1 = f(x_1) = sin(pi/8)-cos(pi/8)`
`x_2 = pi/3, y_2 =f(x_2) = sqrt(3)/2-1/2`

we observe a maximum deviation of `0.007` nearly

Attached a plot with `f(x)` and `y`

The difference between the computed volumes using either function is in the order of `0.0032` units