Question

What is the volume of the solid produced by revolving `f(x)=x^2-x+1, x in [1,3]`around `x=1`?

Answer 1

`(32pi)/3`

Explanation:

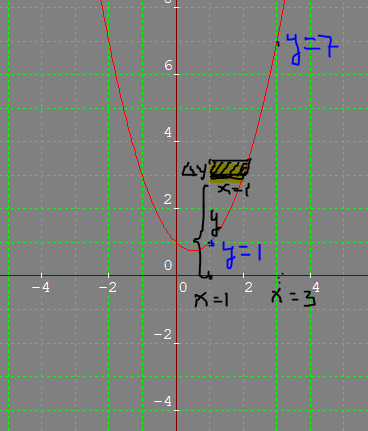
The given function is a parabola opening upwards, as shown in the figure. The portion to revolved lies between x=1 and 3 and the axis of revolution is x=1

Now consider an elementary strip of thickness `Delta y` and length (x-1) at a distance y from the x-axis. On revolving it around x=1, the volume of the solid so generated would be `pi (x-1)^2 Delta y`. Pl see attached figure.

The volume of the whole solid so generated would be

`int_(y=1)^ (y=7) pi (x-1)^2 dy`

From the given eq y= `x^2-x+1` it would be dy= (2x-1)dx. Using this substitution, the volume integral would become

`pi int_(x=1)^(x=3) (x-1)^2 (2x-1)dx`

=`pi int_1^3 (2x^3-5x^2 +4x -1)dx`

=`pi[2x^4 /4 -5 x^3 /3 +4x^2 /2 -x]_1^3`

=`pi[81/2-135/3 +18-3-(1/2 -5/3+2-1)]`

=`pi[81/2 -135/3+15 -3/2 +5/3]`

=`pi[39+15-130/3]` =`(32pi)/3`

Answer 2

The region is not fully specified. I'll answer both possible questions.

Explanation:

`f(x)=x^2-x+1` for `x in [1,3]` around the line `x=1`.

Upper Region
If the region is above the parabola and below `y=7`, then we have:
The region bounded by the functions is shown below in red.
If we take a representative slice parallel to the axis of revolution we have a slice at `x` and thickness of `dx`.

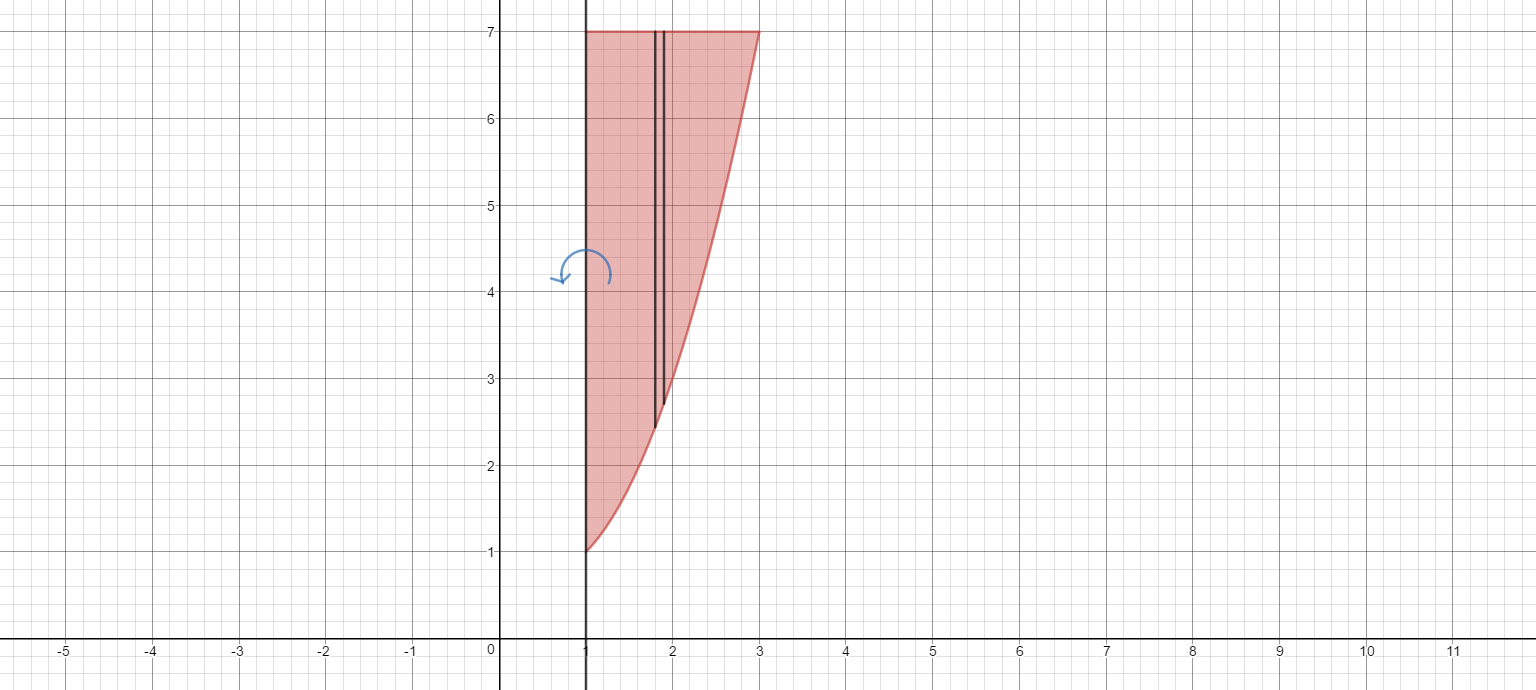
When we revolve the slice, we get a cylindrical shell with volume

`2pirh * "thickness"`

In this case `r = x-1`
(Because we're revolving around `x=1` the radius is the distance between the `x` value of the slice and `1`.)

and `h = 7-(x^2-x+1)`
(The height of the slice is the upper `y` value minus the lower.)

The thickness is `dx`

The volume of the representative shell is: `2pi(x-1)(7-(x^2-x+1))dx`

We will be integrating with respect to `x`, so we observe that `x` varies from `1` to `3`.

The volume of the solid is
`V=int_1^3 2pi(x-1)(7-(x^2-x+1))dx`

`= 2pi int_1^3 (x-1)(7-(x^2-x+1))dx`

`= 2pi(16/3) = (32pi)/3`

LowerRegion
If the region is belowthe parabola and above the `x`-axis, then we have:
The region bounded by the functions is shown below in red.
If we take a representative slice parallel to the axis of revolution we have a slice at `x` and thickness of `dx`.

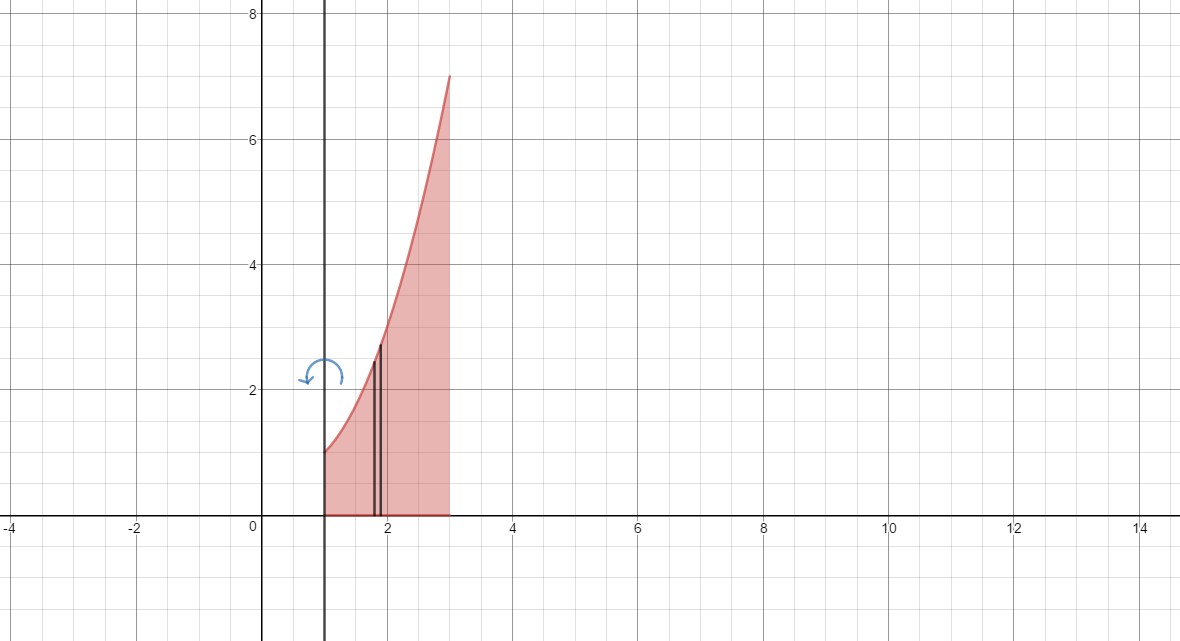
When we revolve the slice, we get a cylindrical shell with volume

`2pirh * "thickness"`

In this case `r = x-1`
(Because we're revolving around `x=1` the radius is the distance between the `x` value of the slice and `1`.)

and `h = (x^2-x+1)`
(The height of the slice is the upper `y` value minus the lower. value, which is `0`)

The thickness is `dx`

The volume of the representative shell is: `2pi(x-1)(x^2-x+1)dx`

We will be integrating with respect to `x`, so we observe that `x` varies from `1` to `3`.

The volume of the solid is
`V=int_1^3 2pi(x-1)(x^2-x+1)dx`

`= 2pi int_1^3 (x-1)(x^2-x+1)dx`

`= 2pi(26/3) = (52pi)/3`