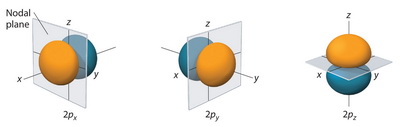
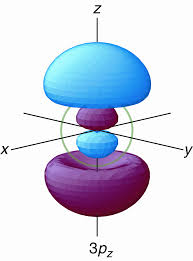
What’s the difference between a p-orbital of the second shell and a p-orbital of the third shell? Thank you!
1 Answer
The number of radial nodes, which are spherical nodal shells.
Recall that
- the principal quantum number
#n# gives the energy level of the orbital. - the angular momentum quantum number
#l# labels the shape of the orbital (#s,p,d,f, . . . # ).
Well, the total number of nodes (regions where electrons cannot be found) is given by
By subtraction, the number of radial nodes (spherical nodal shells) is given by:
#overbrace(n - 1)^"Total nodes" = overbrace((n - l - 1))^"Radial nodes" + overbrace(l)^"Angular nodes"#
So having an orbital of one higher
#bb2p -> n - l - 1#
#= bb2 - 1 - 1 = ul(bb0 " radial nodes")#
#bb3p -> n - l - 1#
#= bb3 - 1 - 1 = ul(bb1 " radial node")#
And this can be visually seen:
Circled in green is the
This is also seen in radial density distributions:
The radial node shows up at the point where the graph dips down to
That shows where the orbital wave function goes to zero (here, close to