Question

What volume of 0.25 M `HCl` solution must be diluted to prepare 1.00 L of 0.040 M `HCl`?

Answer 1

`"160 mL"`

Explanation:

The most important thing to keep in mind when it comes to diluting solutions is that the number of moles of solute must remain constant at all times.

Simply put, the number of moles of solute present in the dilute solution must be equal to the number of moles of solute present in the concentrated sample.

This is the key to any dilution calculation. As you know, molarity is defined as

`color(blue)("molarity" = "moles of solute"/"liters of solution")`

In essence, when you dilute a solution, you decrease its concentration by increasing its volume, which in turn is done by adding more solvent to the solution.

`color(white)(a)`

![)

`color(white)(a)`

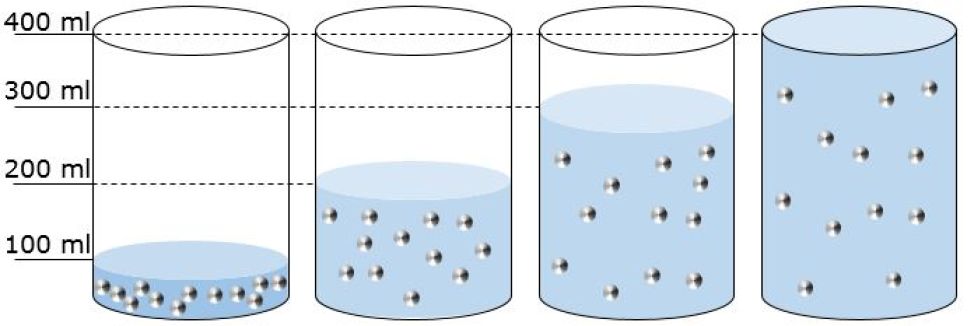
So, you know the molarity and volume of the target solution, which means that you can determine how many moles of hydrochloric acid it must contain

`color(blue)(c = n/V implies n = c * V)`

`n_(HCl) = "0.040 mol" color(red)(cancel(color(black)("L"^(-1)))) * 1.00color(red)(cancel(color(black)("L"))) = "0.040 moles HCl"`

Now your task is to determine what volume of the concentrated solution would contain this many moles of hydrochloric acid.

`color(blue)(c = n/V implies V = n/c)`

Plug in your values to get

`V = (0.040color(red)(cancel(color(black)("moles"))))/(0.25color(red)(cancel(color(black)("mol")))"L"^(-1)) = "0.16 L"`

Expressed in milliliters and rounded to two sig figs, the answer will be

`V = color(green)("160 mL")`

This is exactly what the formula for dilution calculations allows you to do

`color(blue)(overbrace(c_1 xx V_1)^(color(black)("moles of solute in concentrated solution")) = overbrace(c_2 xx V_2)^(color(black)("moles of solute in diluted solution"))`

Here you have

`c_1`, `V_1` - the molarity and volume of the concentrated solution
`c_2`, `V_2` - the molarity and volume of the diluted solution

Once again you will have

`V_1 = c_2/c_1 * V_2`

`V_1 = (0.040color(red)(cancel(color(black)("M"))))/(0.025color(red)(cancel(color(black)("M")))) * "1.00 L" = color(green)("160 mL")`

So, you would prepare this solution by adding enough water to your `"160-mL"` sample of `"0.25-M"` hydrochloric acid solution to make the total volume of the resulting solution equal to `"1.00 L"`.