Question

Which type of transformation does not preserve orientation?

Answer 1

Reflection does not preserve orientation.
Dilation (scaling), rotation and translation (shift) do preserve it.

Explanation:

Perfect example of "oriented" figure on a plane is the right triangle `Delta ABC` with sides `AB=5`, `BC=3` and `AC=4`.

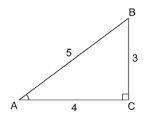
To introduce orientation, let's position ourselves above the plane and, looking down on this triangle, notice that the way from vertex `A` to `B` and then to `C` can be viewed as the clockwise movement.

Rotation, translation (shift) or dilation (scaling) won't change the fact that the direction `A->B->C` is clockwise.

Use now a reflection of this triangle relative to some axis. For instance, reflect it relative to a line `BC`. This transformation will leave vertices `B` and `C` in place (that is, `B'=B` and `C'=C`), but vertex `A` from being to the left of line `BC` will move to the right of it to a new point `A'`.

The way `A'->B->C` is counterclockwise. That is a manifestation of (1) our triangle has orientation and (2) the transformation of reflection does not preserve the orientation.