Question

Why can't the Pythagorean theorem be used to solve an oblique triangle?

Answer 1

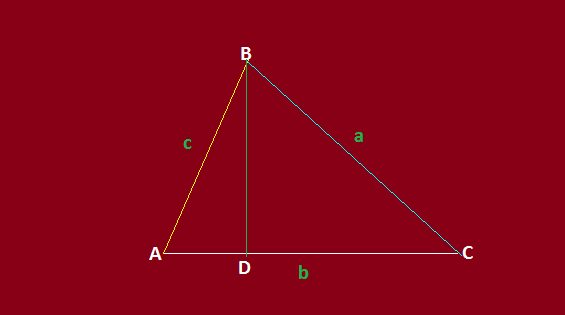
By definition an oblique triangle is any triangle that has no right angle. In the above figure `Delta ABC` is an oblique triangle. So no angle of this triangle is right angle..`BD` is the perpendicular drawn from`B` on `AC`
Pythagorean theorem relates three sides of a triangle . According to this theorem , the square of hypotenuse, the largest side of the right angled triangle is equal to the sum of squares of other two sides of the triangle,

In the above figure applying Pythagorean theorem for `Delta BDC and Delta BDA` we can write

`BC^2=BD^2+CD^2.......[1]`

`AB^2=BD^2+AD^2.......[2]`

From equation [1]

`BC^2=BD^2+(AD-AC)^2`

`=>BC^2=BD^2+AD^2+AC^2-2ADxxAC`

`=>BC^2=AB^2+AC^2-2(AD)/(AB)xxABxxAC`

`=>a^2=c^2+b^2-2cosAxxcxxb`

`=>a^2=c^2+b^2-2bc cosA.........[3]`

Similarly we can establish

• `b^2=c^2+a^2-2ac cosB...........[4]`
• `c^2=a^2+b^2-2ab cosC............[5]`

So in general the every relation ([3],[4],[5]) among three sides is always associated with cosine of one of the angle of the triangle if the triangle is oblique one. When an angle `A or B or C` becomes right angle then the value of `cosA or cosB or cosC` becomes ZERO and consequently the relation becomes the relation that is obtained from Pythagorean theorem.