Question

Will the reaction `Ag + CuNO_3` occur?

Answer 1

No. Since `"Cu"` is more active than `"Ag"`, it is disfavored for this reaction to occur, as copper would more rather be oxidized than silver would (and thus copper would less rather be reduced than silver would).

---

This is supposed to be a redox (oxidation-reduction) reaction, where the intention is to oxidize `"Ag"(s)` and reduce `"Cu"^(+)(aq)`.

The half-reactions are:

`"Ag"(s) -> "Ag"^(+)(aq) + e^(-)`, `E_"oxid"^@ = -"0.80 V"`

`"Cu"^(+)(aq) + e^(-) -> "Cu"(s)`, `E_"red"^@' = ???`

so the reaction would hypothetically be

`color(red)("Ag"(s) + "CuNO"_3(aq) -> "Cu"(s) + "AgNO"_3)`.

WHERE IS THE REDUCTION POTENTIAL FOR COPPER(I)?

For the copper reaction, I actually can't find data for its standard reduction potential, but I can work around that.

The reference above shows the following, which we can add together:

`"Cu"^(+)(aq) -> cancel("Cu"^(2+)(aq)) + cancel(e^(-))`, `E_"oxid"^@ = -"0.15 V"`
`cancel("Cu"^(2+)(aq)) + cancel(2)^(1)e^(-) -> "Cu"(s)`, `E_"red"^@ = "0.34 V"`
`"--------------------------------------"`
`"Cu"^(+)(aq) + e^(-) -> "Cu"(s)`,

`E_"red"^@' = E_"red"^@ + E_"oxid"^@`

`= 0.34 + (-0.15) = "0.19 V"`

So, the final standard cell potential for the overall redox reaction would be:

`E_"cell"^@ = E_"red"^@' + E_"oxid"^@`

`= 0.19 + (-0.80) = color(blue)(-"0.61 V")`

...a negative number.

WHAT DOES A NEGATIVE STANDARD CELL POTENTIAL MEAN?

Note the following equations, which helps us determine whether a negative `E_"cell"^@` is spontaneous or not.

`color(blue)(DeltaG^@ = -nFE_"cell"^@)`

`color(blue)(DeltaG = DeltaG^@ + RTlnQ)`

where:

• `DeltaG` is the general Gibbs' free energy and `DeltaG^@` is the standard Gibbs' free energy.
• `n` is the number of electrons transferred in the overall reaction. In this case it is `1`.
• `F` is the Faraday constant, which is about `"96485 C/mol"`.
• `E_"cell"^@` is the standard cell potential, which we have for our reaction to be negative.

Normally, `DeltaG` tells us spontaneity at any temperature (spontaneous if negative, nonspontaneous if positive, equilibrium if `0`).

But since standard reduction potentials are defined for `T = 25^@ "C"`, `P = "1 atm"` (or `"1 bar"`, depending on how new your book is), and concentrations of `"1 M"`, we can say that `\mathbf(Q = K = 1)`.

Thus, with `RTlnQ = RTln(1) = 0`, `DeltaG = DeltaG^@`, so we really do have in this case, `DeltaG^@` telling us about the spontaneity of this particular reaction at specifically `25^@ "C"`.

Now we can plug into the first equation to get

`color(green)(DeltaG = -nFE_"cell"^@)`.

Since `E_"cell"^@ < 0`, `F > 0`, and `n > 0`, `\mathbf(DeltaG > 0)` and the reaction is nonspontaneous. Therefore, it doesn't readily occur without some sort of outside "push".

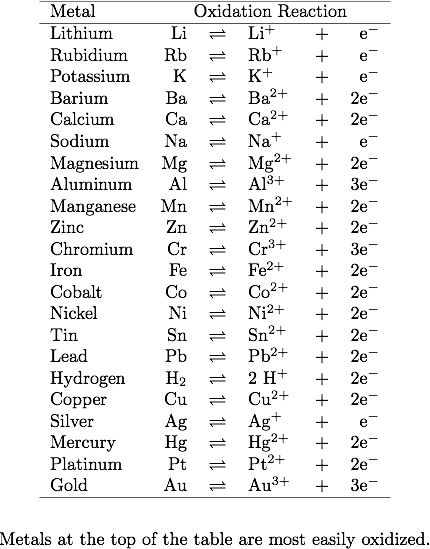
This is consistent with the activity series, which tells us that copper is more easily oxidized than silver, and thus, would rather be oxidized than reduced.