How do you use a graph to show that the limit does not exist?
1 Answer
Sep 27, 2014
Remember that limits represent the tendency of a function, so limits do not exist if we cannot determine the tendency of the function to a single point. Graphically, limits do not exist when:
- there is a jump discontinuity
(Left-Hand Limit#ne# Right-Hand Limit)
The limit does not exist at#x=1# in the graph below.
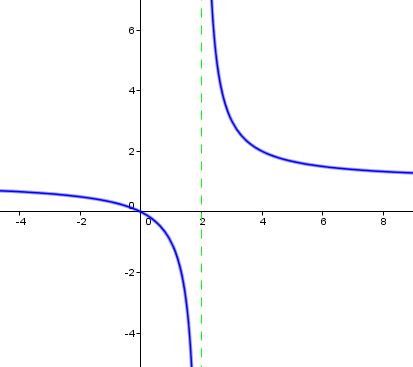
- there is a vertical asymptote
(Infinit Limit)
(Caution: When you have infinite limits, limits do not exist.)
The limit at#x=2# does not exist in the graph below.
- there is a violent oscillation
(e.g.,#sin(1/x)# at#x=0# , shown below)

I hope that this was helpful.

