On a ship sailing north, a woman notices that a hotel on a shore has a bearing of 20. A little while later, after having sailed 40km, she observes that the bearing of the hotel is now 100. How far is the ship from the hotel?
1 Answer
Always draw a sketch of the problem with all your angles and distances labeled correctly.
Explanation:
Here is a sketch of the problem:
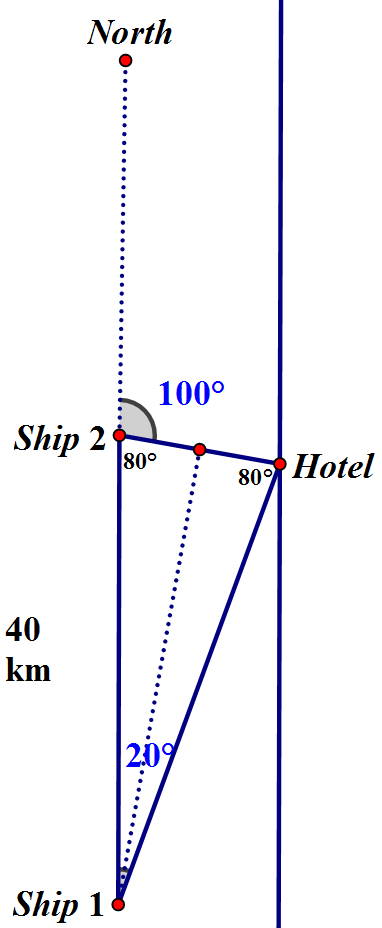
The word "bearing" is always clockwise with respect to North. So, at time 1, the hotel is 20 degrees clockwise from North. Similarly, at time 2 the hotel is 100 degrees clockwise from North.
The ship is traveling parallel to the shoreline, so use what you know about straight lines, transversals and parallel lines to fill in the remaining degrees shown in the sketch above.
Because we know the base angles of the triangle are both 80 degrees, the triangle must be isosceles. This means both the distance traveled from time 1 to time 2 is 40 km, but also the opposite side of the triangle must be 40 km (def'n of isosceles).
Next, draw an angle bisector that bisects the 20 degree angle and will be perpendicular to the base of the triangle. This will form two congruent right triangles .
Now, we can use trigonometry to solve for the length of the base for each of the right triangles:
Finally, we can determine the distance from the ship at time 2 and the hotel:
distance =

