Question

Is a rhombus a square?

Answer 1

In general, no.

Explanation:

Recall the two definitions:

• A square is a quadrilateral that has four equal sides and four equal angles.
• A rhombus is a quadrilateral that has four equal sides.

So, a quadrilateral that is a square is always a rhombus, because a square has four equal sides.
But the converse is not true in general, because the definition of rhombus doesn't involve angles equality. Hence the only rhombi that are squares are the rhombi that satisfy also the condition on angles. Precisely, they are all the squares.

We can the affirm that the set of squares is a subset of the set of rhombi.

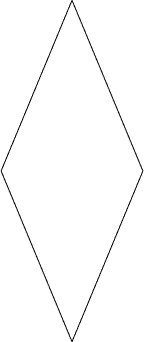
An example of rhombus that it's not a square is a rhombus `ABCD` in which `AB=BC=CD=AD=1` and the angles are `hat A = hat C = 45^@` and `hat B = hat D = 135^@`.
A picture of such a rhombus is the following one: