How do you find the Maclaurin series for #ln(2-3x) #?
2 Answers
Explanation:
Using the Maclaurin series ln (1-X)=-X-X^2/2-X^3/3-...-X^n/n-...,#
Let
#=ln2 - sum_(n=1)^(∞) (3/2)^(n)/(n!) x^n#
Explanation:
In order to find such Maclaurin series, which is just a special case of a Taylor series centered at
Remember that a Maclaurin series can be expressed in the following way:
# = sum_(n=0)^(∞) f^(n)(0)/(n!) x^n#
Let
Calculating our derivatives:
#f^(1)(x) = - (3x)/(2-3x)#
# = -3(2-3x)^(-1) #
#f^(2)(x) = 3(2-3x)^(-2)(-3)#
#= -9(2-3x)^(-2)#
#f^(3)(x) =18(2-3x)^(-3)(-3)#
#= -54(2-3x)^(-3)#
#f^(4)(x) =162(2-3x)^(-4)(-3)#
#= -486(2-3x)^(-4)#
Since a Maclaurin series is centered at
#f(0) = 0#
#f^(1)(0) = -3/2 #
#f^(2)(0) = -9/4#
#f^(3)(0) = -54/8 #
#f^(4)(0) = -486/16 #
Now that we have our derivatives, we can simply substitute them into our infinite sum:
#=ln2 - sum_(n=1)^(∞) (3/2)^(n)/(n!) x^n#
Notice that our infinite sum does not start at
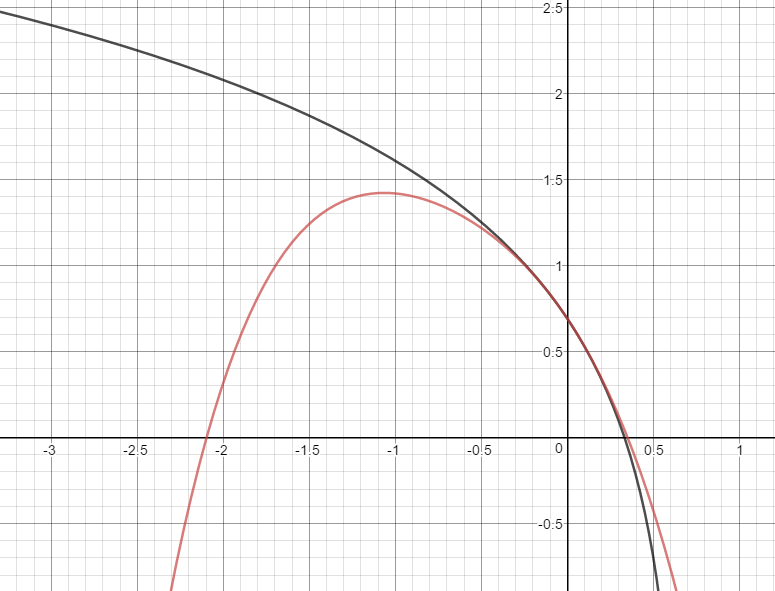
To get an idea for how this can be visualized - here are the graphs of each function (the original and the approximation).
Graph of
graph{ln(2-3x) [-10, 10, -5, 5]}
Graph of
graph{ln 2 - (3/2)^(1)/(1!) x - (3/2)^(2)/(2!) x^2 - (3/2)^(3)/(3!) x^3 - (3/2)^(4)/(4!) x^4 [-10, 10, -5, 5]}
When both overlap, we can see that the approximation looks to be correct.