Question

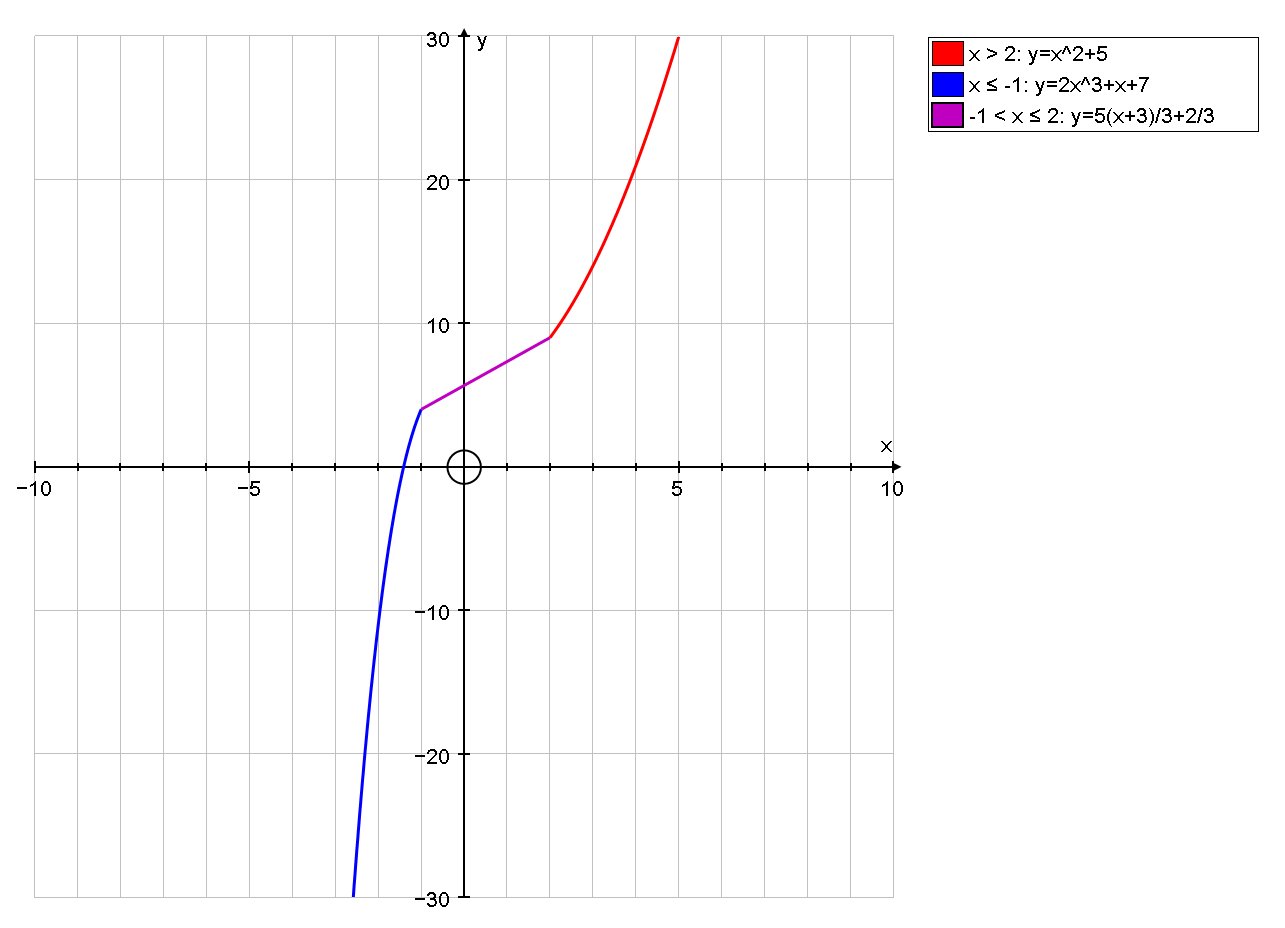
How do we find the values of k and m that makes function continue anywhere piecewise function of `(x^2) + 5` when x > 2, `m(x+3) + k` when `-1 < x <=2` and `2(x^3) + x + 7` when `x <=-1`?

Answer 1

`m = 5/3`
`k = 2/3`

Explanation:

Here is an example of a continuous function.

Here is an example of a discontinuous function.

So, continuous functions are where everything is connected; there are no gaps or holes.

The y-value of `x^2 + 5` when `x =2`, (even though this would be a hole) is `9`.

We need the graph of `y= m(x + 3) +k` to end at the point `(2,9)`.

The `y-` value of `y = 2x^3 + x + 7` at `x = -1` is `y = 2(-1)^3 - 1 + 7 = -2 - 1 + 7 = 4`.

We need the graph of `y = m(x + 3) + k` to start at the point `(-1, 4)`.

Hence, we can write a systems of equations with respect to `m` and `k`, since we know two inputs/outputs.

`4 = m(-1 + 3) + k`
`9 = m(2 + 3) + k`

`k = 9 - 5m`

`4 = 2m + 9 - 5m`

`-5 = -3m`

`m = 5/3`

`9 = 5m + k`

`9 = 5(5/3) + k`

`9 = 25/3 + k`

`k = 2/3`

So, the function in the interval `-1 < x ≤ 2` is `y = 5/3(x + 3) + 2/3`.

Answer 2

`m=5/3` and `k=2/3`,

`f(x)={ (x^2+5,x>2), (5/3(x+3)+2/3,-1 < x <= 2), (2x^3+x+7,x<=-1) :}`

Explanation:

We want to find `m` and `k` such that `f(x)` is continuous:

`f(x)={ (x^2+5,x>2), (m(x+3)+k,-1 < x <= 2), (2x^3+x+7,x<=-1) :}`

Just think about what we know so far, and how it would look:

When `x=-1 => f(-1)=2(-1)^3-1+7=-2-1+7=4`
When `x=2 => f(2)=2^2+5=4+5=9`

So for the mid interval we need a straight line whose equation is `y=m(x+3)+k` which passes through `(-1,4)` and `(2,9)`

This line would have the following gradient:
`m=(Delta y)/(Delta x) = (9-4)/(2-(-1)) = 5/3`

So our required line passes through `(-1,4)` (equally we could you the other coordinate and get the same answer) and has gradient `m=5/3`, so using `y=m(x+3)+k` we have:

`5/3(-1+3) + k = 4`
`5/3(2) + k = 4`
`10/3 + k = 4`
`k = 4=10/2 =2/3`

So the mid-section has equation `m(x+3)=k` with `m=5/3` and `k=2/3`,

ie `y=5/3(x+3)+2/3`,Which we can graph to confirm