the area of the polar curve given by:
#color(red)[A=1/2int_(theta_1)^(theta_2)r^2*d(theta)#
The interval of the integral #theta in [pi/8,pi/4]#
now lets setup the integral in our interval:
#A=1/2int_(pi/8)^(pi/4)(8sin(3theta-(2pi)/4) +4theta)^2*d(theta)#
#A=1/2int_(pi/8)^(pi/4)(4theta-8cos(3theta))^2*d(theta)#
#A=1/2int_(pi/8)^(pi/4)(4theta-8cos(3theta))^2*d(theta)#
#A=int_(pi/8)^(pi/4)[8(theta)^2-32thetacos(3theta)+32cos^2(3theta)]*d(theta)#
#=[(24sin(6theta)-96xsin(3theta)-32cos(3theta)+24theta^3+144theta)/9]_(pi/8)^(pi/4)#
#[(3*2^(21/2)pisin((3pi)/8)+2^(27/2)*cos((3pi)/8)+21*2^(5/2)pi^3+(9*2^(19/2)-6144)pi-3*2^(23/2)+2048)/(9*2^(17/2))]=4.68224#
Approximation:
#A=4.68224#
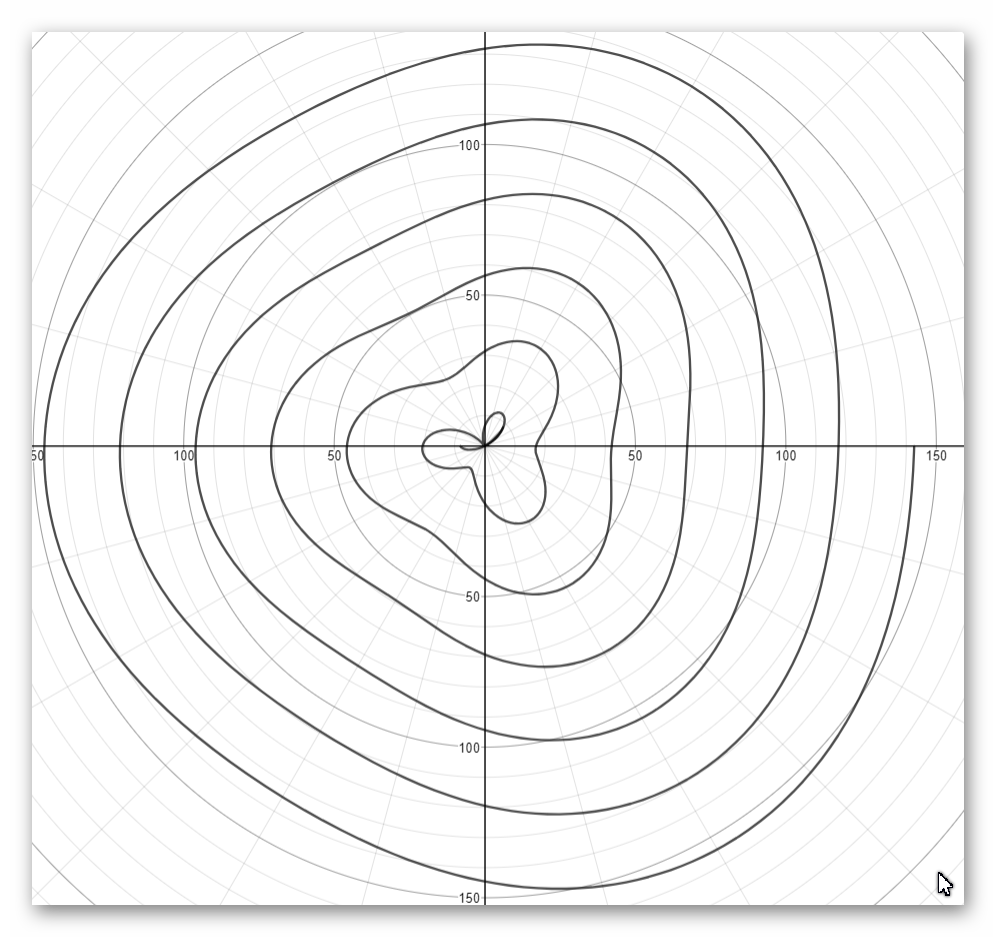
see below the sketch of the polar curve:
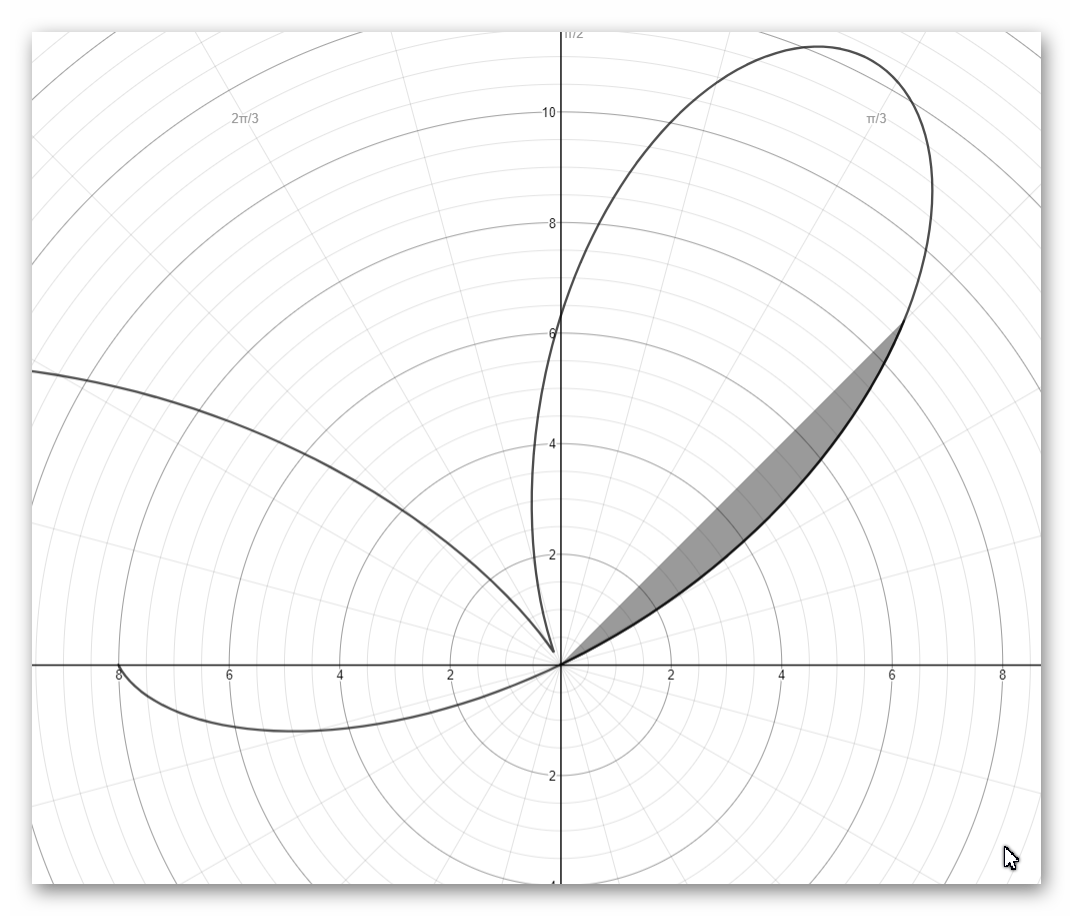
see below the region bounded by the curve from #pi/8# to#pi/4#
#"I hope that helpful"#



