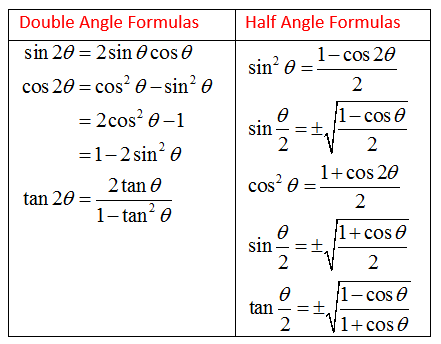
How do you simplify #f(theta)=2cot(theta/2)-csc(theta/4)-cos(theta/4)# to trigonometric functions of a unit #theta#?

1 Answer
sankarankalyanam
Jul 24, 2018

#color(indigo)(f(theta) = (2 * pm sqrt((1 + cos theta) / (1 - cos theta))) - pm 1 / (sqrt(1/2 (1 - pm sqrt(1/2 (1 - cos theta))))) - pm sqrt(1/2 (1 pm sqrt(1/2 (1 + cos theta)))#

Explanation:

https://www.onlinemathlearning.com/double-angle-formula.html

#f(theta) =2 cot (theta/2) - csc (theta/4) - cos (theta/4)#

#color(brown)(cot (theta/2) = 1 / tan (theta/2) = pm sqrt((1 + cos theta) / (1 - cos theta))#

#sin (theta/4) = pm sqrt(1 /2 (1 - sin (theta/2)))#

#color(brown)(csc (theta/4) = 1 / sin (theta / 4) = pm 1 / (sqrt(1/2 (1 - pm sqrt(1/2 (1 - cos theta)))#

#cos (theta/4) = pm sqrt(1 /2 (1 + cos (theta/2)))#

#color(brown)(cos (theta / 4) = pm sqrt(1/2 (1 pm sqrt(1/2 (1 + cos theta)))#

#color(indigo)(f(theta) = (2 * pm sqrt((1 + cos theta) / (1 - cos theta))) - pm 1 / (sqrt(1/2 (1 - pm sqrt(1/2 (1 - cos theta))))) - pm sqrt(1/2 (1 pm sqrt(1/2 (1 + cos theta)))#

Related questions
See all questions in Half-Angle Identities
Impact of this question
2611 views around the world
You can reuse this answer
Creative Commons License