Question

Question #f3e7d

Answer 1

`4`

Explanation:

The first thing to do here is to determine how many lattice points you get for a face-centered cubic unit cell.

For starters, all three types of cubic lattice unit cells, i.e. simple cubic, body-centered cubic, and face-centered cubic, have lattice points in the corners of the cubic unit cell.

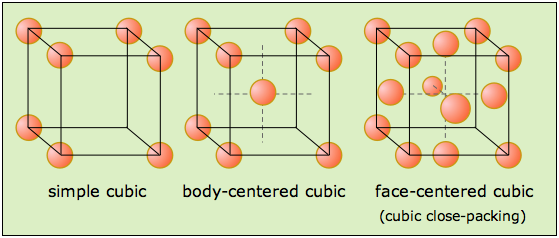
So right from the start you know that the face-centered cubic cell has at least `8` lattice points, one for each corner of the cube.

Now, the characteristic of a face-centered cubic cell is that it also contains lattice points in the centers of the cube's faces.

Since a cube has a total of six faces, it follows that the total number of lattice points you get per unit cell is

`"no. of lattice points" = overbrace("8 lat. pts.")^(color(blue)("corners")) + overbrace("6 lat. pts.")^(color(red)("faces")) = "14 lat. pts."`

To determine the number of atoms that you can fit into a face-centered cubic unit cell, you need to look at how the lattice structure is arranged.

For a given unit cell, pick one of its corners. This corner is being shared by a total of eight unit cells. This means that each corner in a unit cell will contain `1/8"th"` of an atom.

Simply put, eight unit cells share one atom per corner.

For a given cell, pick one of its faces. This face is being shared by a total of two unit cells, which means that each face in a unit cell will contain `1/2` of an atom.

In other words, two unit cells share one atom per face.

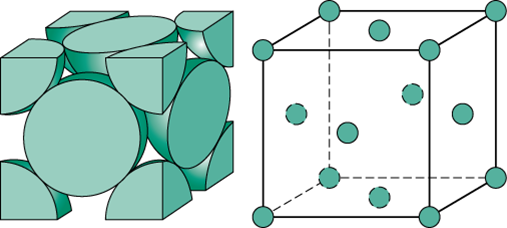
So, the total number of atoms that can fit into a face-centered cubic unit cell will be

`"no. of atoms" = overbrace( 1/8 xx 8)^(color(blue)("8 corners")) + overbrace(1/2 xx 6)^(color(red)("6 faces")) = color(green)("4 atoms")`