Question

Question #f2a5b

Answer 1

Don't let the language scare you. This is actually solvable on general chemistry principles, once we distill this down to something more understandable.

I will show you that most jargon aside, this question is really asking:

"What is the wavelength corresponding to the minimum energy needed to excite an electron from the valence band to the conduction band?"

---

GERMANIUM AS AN INTRINSIC SEMICONDUCTOR

According to Band Theory, since `"Ge"` metal has a band gap near or less than about `"1 eV"`, it can function as a good semiconductor (Integrated Electronics, Milman and Halkias, pg. 16).

Because its conductivity increases with temperature, it is an intrinsic semiconductor, requiring no dopant (such as silicon).

It has an energy gap (`"0.72 eV"`) separating its valence band (lower in energy) and its conduction band (higher in energy), called the forbidden energy gap.

![)

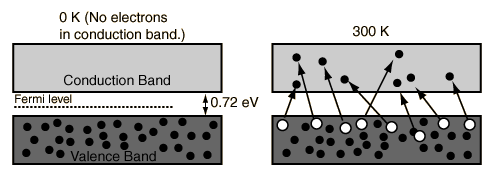
At the Fermi level, which commonly is right around the HOMO-LUMO gap at `"0 K"`, we basically have a bunch of electrons in the valence band that are on the verge of being promoted to the conduction band.

Raise the temperature a little, or add a bit of energy (say, `"0.72 eV"`), and you can excite an electron past this "forbidden energy gap" in germanium and let it conduct electrical current.

SOLVING FOR THE MAXIMUM WAVELENGTH

We know that wavelength `lambda = 1/nu`, and the frequency `nu prop E`, the energy. That means `E prop 1/lambda`, and the "maximum `lambda`" is the minimum `E`.

Next, `"Ge"` has two valence electrons in its `4p` orbitals. When one of them is excited, band theory postulates that it leaves behind a positively-charged "hole".

So, asking how to "generate an electron-hole pair" simply says:

"How does one annihilate one of the two electrons, and then create an excited one in a higher-energy, previously-unoccupied orbital?"

Thus, the question is really asking:

"What is the wavelength corresponding to the minimum energy needed to excite an electron from the valence band to the conduction band?".

Hence, we should solve the following equation from general chemistry:

`\mathbf(E = hnu = (hc)/lambda)`

Now that we've clarified the language, this is pretty easy, no? No unit conversion necessary.

`color(blue)(lambda) = (hc)/E`

`= (12400 cancel("eV")cdot"Å")/(0.72 cancel("eV"))`

`=` `color(blue)("17222 Å")`

This web page seems to agree with me.