Question

How and when do we use SOHCAHTOA?

Answer 1

Below are a few examples I have prepared.

Explanation:

Multiplication:

Example: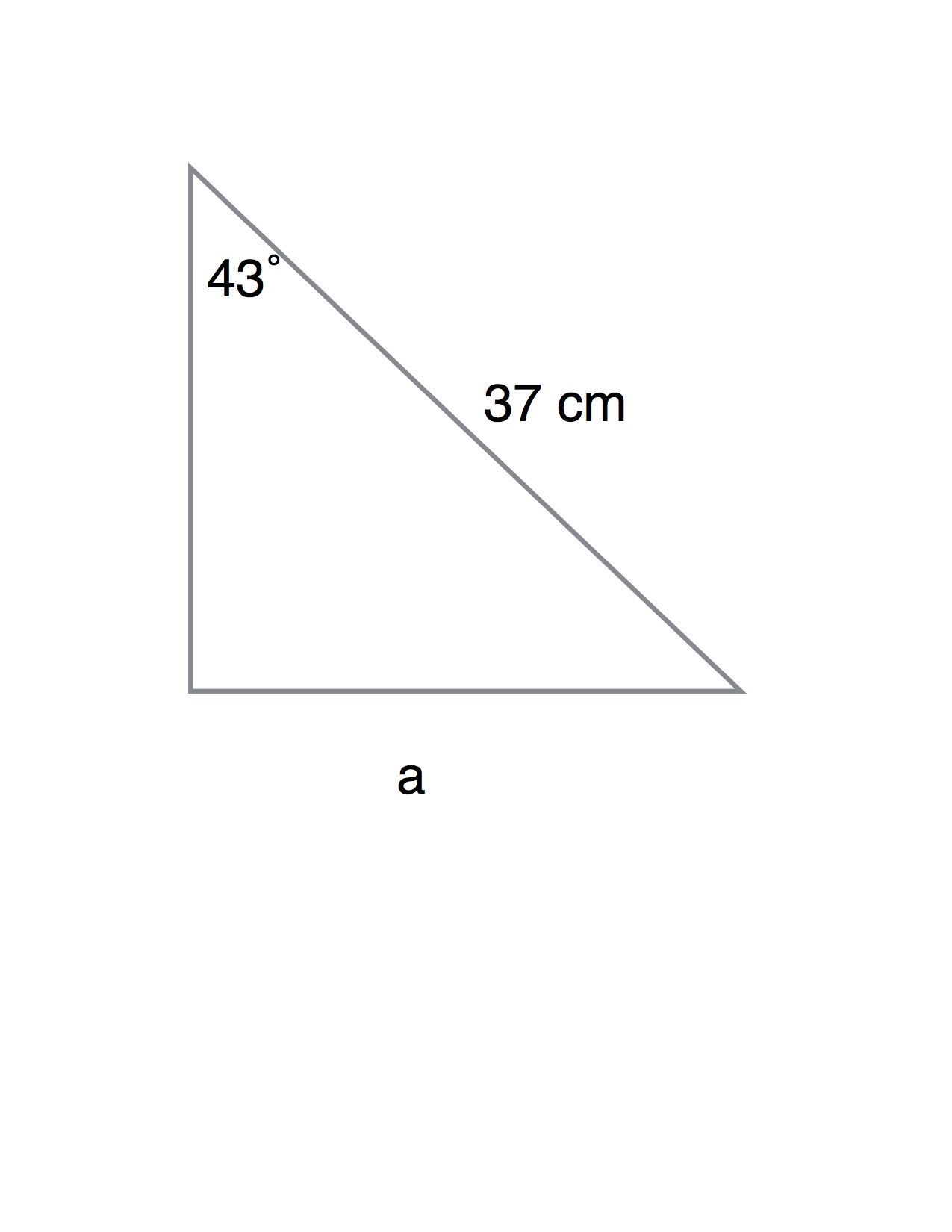

First, we have to find what positions side a and the side measuring 37 cm are relative to the given angle, which is `43˚`.

`•`a is opposite

`•` 37 cm is the hypotenuse

By SOHCAHTOA, an opposite and a hypotenuse gives `sin`.

Now, we write our proportion.

`a/37 = sin43/1`

Now, we must solve for a. This can be done by using the property `a/b = n/m -> a xx m = b xx n`

`a xx 1 = 37(sin43)`

`a = 37sin43`

Calculating, we get that a measures 25.23 cm.

The trick to the multiplication: always make a proportion.

Division:

Consider the following example:

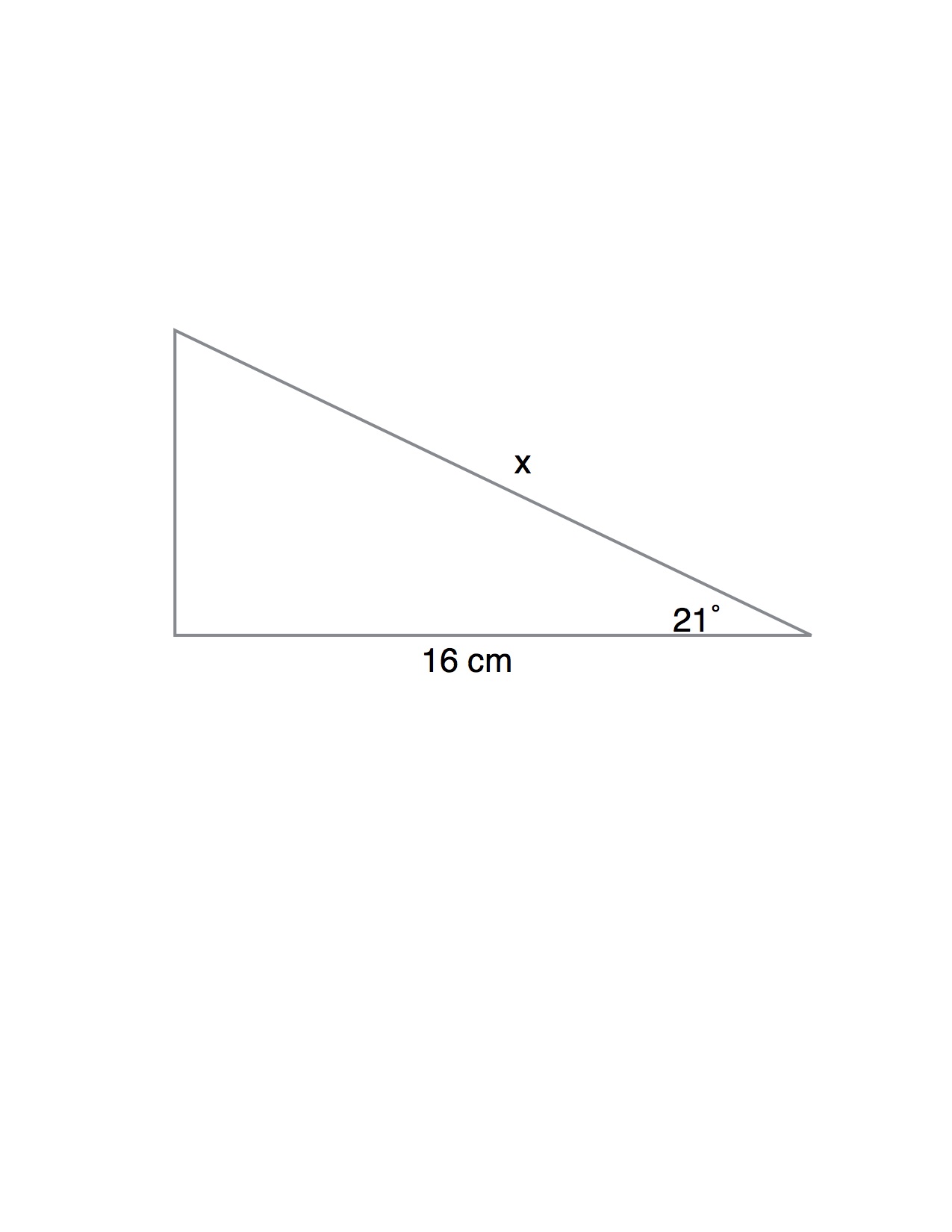
Once again, from `21˚`, identifying the sides we know, we find that we know adjacent and hypotenuse. Looking through SOHCAHTOA, we find that adjacent/hypotenuse is represented by `cos`.

Writing our proportion:

`16/x = (cos21˚)/1`

Here, we have a division. Same thing as last time however; we use the property `a/b = m/n -> a xx n = b xx m`

`x = 16/(cos21˚)`

`x = 17.14 cm`

The best trick:

If you are solving for the hypotenuse, and the ratio you are using is sin or cos, then it will be a division (the side you are solving for is on the bottom ). If you are solving for one of the legs, it will be a multiplication. However, tangent depends on the case (it can be either way). As I said many times before, most importantly, set up your proportion and use the property `a/b = m/n -> a xx n = b xx m`: This is key to your success in this unit.

Feel free to send me a message if you need any additional help.

Hopefully you understand better now.