A gamma ray has a frequency of #2.88 * 10^21# Hz. What does this mean?
1 Answer
That means it goes through
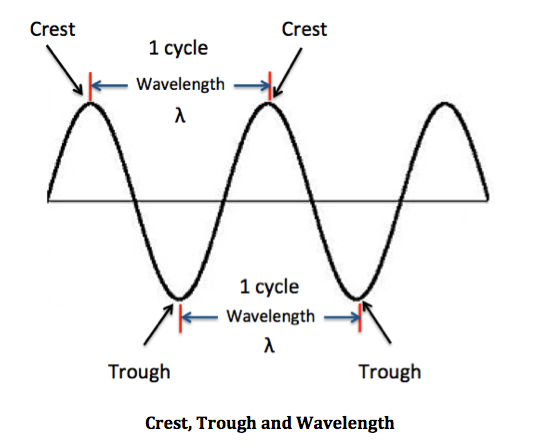
One cycle is any of the following:
- from one crest/antinode to the next crest/antinode
- from one trough to the next trough
- from one node (zero-crossing) to the next node
The equation relating frequency to wavelength and speed is:
#color(blue)(upsilon = lambdanu)# Hence, the speed
#upsilon# of a wave is the wavelength#lambda# of the wave multiplied by its frequency#nu# . If we say wavelength is in#"nm"# , then the speed is in#"nm" xx "1/s" = \mathbf("nm/s")# .
The frequency, then, is really just a measure of how fast the wave is propagating forward irrespective of knowing the wavelength.
You can further realize that we have the following equation:
#color(blue)(E = hnu)# where we should not confuse
#upsilon# with#nu# .#upsilon# is speed, while#nu# is frequency (I didn't use#f# because then I would have to redefine#nu = f# ).
We can then see that a higher

