Question

A helicopter rises at the rate of 8 feet per second from a point on the ground 60 feet from an observer. How do you find the rate of change of the angle of elevation when the helicopter is 25 feet above the ground?

Answer 1

`((d theta )/dt)=96/845 approx .11361` radians/second

Explanation:

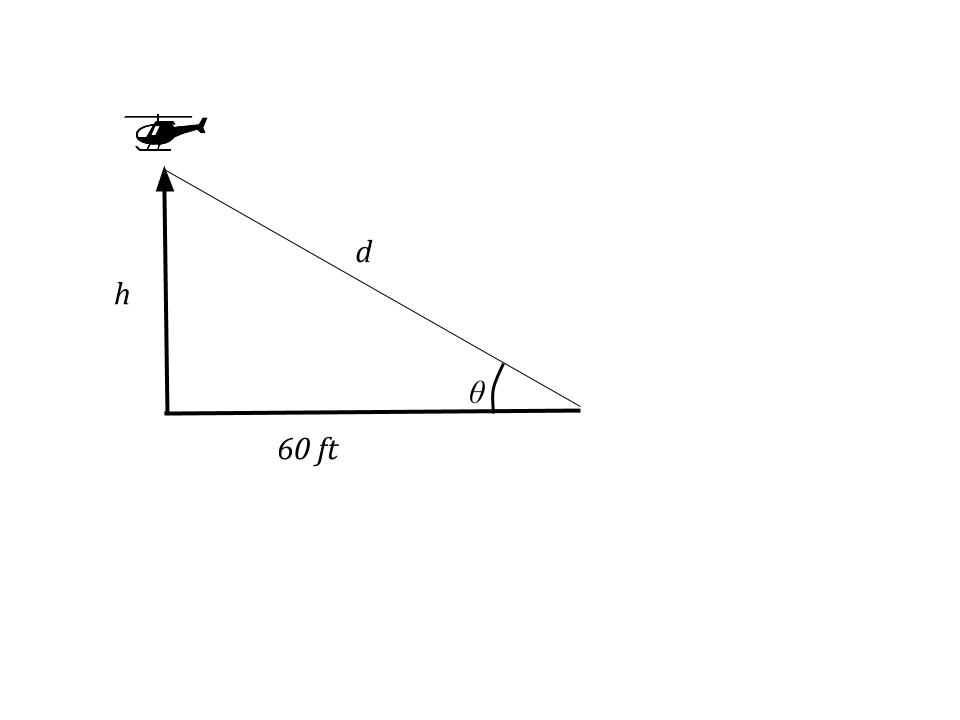
(Drawing above is not to scale)

We know that `(dh)/dt = 8` ft/sec
First, make an equation using the information you have and the variables you want:
`tan theta=h/60`
Now implicitly differentiate with respect to time:
`(d theta )/dtsec^2theta = 1/60(dh)/dt`
Now isolate `(d theta)/dt`:
`(d theta)/dt = (1/60)((dh)/dt)(1/(sec^2theta))`
`(d theta)/dt = (1/60)((dh)/dt)(cos^2theta)`
Now substitute in values that we know:
`(d theta)/dt = (1/60)(8` ft/sec`)(cos^2theta)`

To find `cos^2theta`, we need to know what the distance from the helicopter to the observer is (labeled d in above drawing) using the Pythagorean theorem. We want to know what d is equal to when h is 25:
`60^2+25^2=d^2`
`d=sqrt(3600+625)`
`d=sqrt4225=65` ft from observer to helicopter when helicopter is 25 ft high.

Now, continue simplifying the above equation:
`(d theta)/dt = (1/60)(8` ft/sec`)(cos^2theta)`
`(d theta)/dt = (1/60)(8` ft/sec`)(60/65)^2`
`(d theta)/dt = (1/60)(8)(144/169)`
`(d theta)/dt = 96/845 approx .11361` radians per second