A triangle has corners A, B, and C located at #(1 ,1 )#, #(6 ,8 )#, and #(7 ,4 )#, respectively. What are the endpoints and length of the altitude going through corner C?

1 Answer
yumean1119
Oct 15, 2017

Endpoint: #(329/74, 431/74)#
Height: #27/sqrt(74)#

Explanation:

Here is a graph.
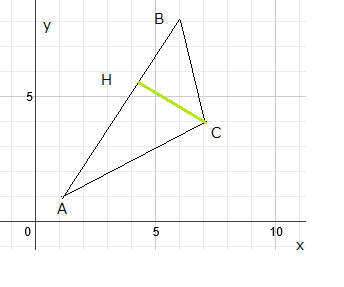
enter image source here

What we want to know is the endpoint(#H#) and length(#CH#) of the altitude.

[Step1: determine the line #AB#]
The slope of line #AB# is #(8-1)/(6-1)=7/5# and the equation is
#y-1=7/5(x-1)#
#y=7/5x-2/5#.

[Step2: determinte the line #CH#]
If the two lines with slope #m_1# and #m_2# cross at the right angle, #color(red)(m_1m_2=-1)# is needed.
Thus, the slope of line CH is #-1/(7/5)= -5/7#.
Its equation is #y-4=-5/7(x-7)#. i.e. #y=-5/7x+9#.

[Step3: calculate the endpoint and height]
Solving the system equations
#y=7/5x-2/5#
#y=-5/7x+9#
and the endpoint #H# is #H(329/74, 431/74)# or, approximately #(4.4459,5.8243)#.

The length of CH is calculated below:
#CH=sqrt((7-329/74)^2+(4-431/74)^2)#
#=27/sqrt(74)#
#=3.1387…#

Related questions
See all questions in Altitudes
Impact of this question
1398 views around the world
You can reuse this answer
Creative Commons License