Question

A triangle has corners at points A, B, and C. Side AB has a length of `12`. The distance between the intersection of point A's angle bisector with side BC and point B is `7`. If side AC has a length of `21`, what is the length of side BC?

Answer 1

BC=19.25

Explanation:

Here is a sketch (not to scale)

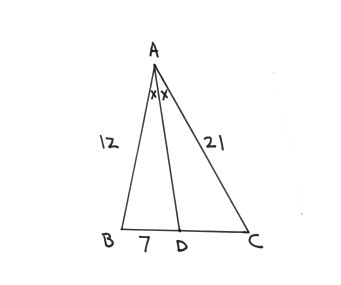
To calculate the length of BC ,we require to find the length of DC.

This can be done by using the #color(blue)" Angle bisector theorem"#

For triangle ABC this is.

`color(red)(|bar(ul(color(white)(a/a)color(black)((BD)/(DC)=(AB)/(AC))color(white)(a/a)|)))`

Substitute the appropriate values into the ratio.

`rArr7/(DC)=12/21`

now cross-multiply

`rArrDCxx12=7xx21rArrDC=(7xx21)/12=12.25`

Thus BC = BD + DC = 7 + 12.25 = 19.25