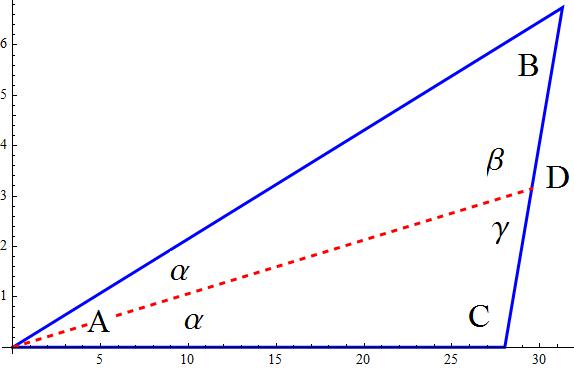
A triangle has corners at points A, B, and C. Side AB has a length of #15 #. The distance between the intersection of point A's angle bisector with side BC and point B is #12 #. If side AC has a length of #27 #, what is the length of side BC?
1 Answer
Aug 12, 2016
Explanation:
Applying sinus law to triangles
and
but
dividing the firts and last equation in both sides we have now
Finally