Question

A triangle has sides A, B, and C. The angle between sides A and B is `(5pi)/12` and the angle between sides B and C is `pi/12`. If side B has a length of 21, what is the area of the triangle?

Answer 1

`color(brown)(" Area of Triangle " A_t = (1/2) a c = 55.16 "sq units"`

Explanation:

![https://.commons.wikimedia.org/wiki/File:Triangulo_rectangulo.PNG]
(useruploads.socratic.org)

`hat C = (5pi)/12, hatA = pi/12, hat B = pi - ((5pi)/12 + pi/12) = pi/2, b = 21`

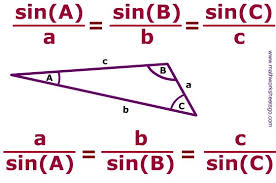
According to Law of Sines,

`a / sin (pi/12) = 21 / sin (pi/2) = c / sin ((5pi)/12)`

`a = 21 sin (pi/12) = 5.44`

`c = 21 sin ((5pi)/12) = 20.28`

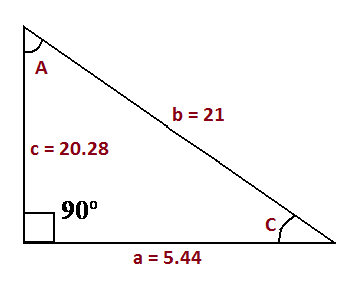
Area of Triangle " A_t = (1/2) a c = (1/2) * 5.44 * 20.28 = 55.16 "sq units"#