Question

Assuming human skin is at 98.6 degrees Fahrenheit, what wavelength is the peak in the human thermal radiation spectrum? What type of waves are these?

Answer 1

`lambda_(max)=9.34 mu m` which is called infrared light.

Explanation:

Wien's displacement law states that the black-body radiation curve for different temperatures peaks at a wavelength inversely proportional to the temperature.

`lambda_(max)=b/T`

where b is Wien's displacement constant, equal to `2.898×10^(−3) m K` and `T` is the temperature in Kelvin. The temperature we were given needs to be converted to Kelvin:

`T = (T_F-32^oF)*(5K)/(1^oF) + 273K = 310.15K`

plugging this into our equation, we get the peak wavelength of radiated light:

`lambda_(max)=(2.898×10^(−3) m K)/(310.15K)=9.34x10^-6m=9.34 mu m`

This is in the range of what is called infrared radiation.

Answer 2

Should be the infrared fingerprint region.

---

We have a relationship for this called Wien's Displacement Law:

`\mathbf(lambda_max = b/T)`

where:

• `b = 2.89777xx10^(-3)` `"m"cdot"K"` is a proportionality constant, probably experimentally determined.
• `T` is temperature in `"K"`.
• `lambda_max` is the wavelength that you observe at its largest spectral energy density.

The spectral energy density is depicted in the following diagram, with respect to wavelength in `"nm"`:

You can think of the spectral energy density as being proportional to the contribution of each wavelength range to some final observed color at a particular temperature. You can see that the peaks would correspond to `lambda_max`.

Converting temperature to `"K"`, we get:

`(98.6^@ "F" - 32) xx 5/9 = 37^@ "C"`

`37 + 273.15 ~~ color(green)("310.15 K")`

And now we get a max wavelength of:

`lambda_max = (2.89777xx10^(-3) "m"cdotcancel"K")/("310.15" cancel"K")`

`= 9.343xx10^(-6) "m"`

Converting this to `mu"m"`, we get:

`= 9.343xx10^(-6) cancel("m") xx (10^6 mu"m")/(1 cancel"m")`

`= color(blue)(9.343)` `color(blue)(mu"m")`

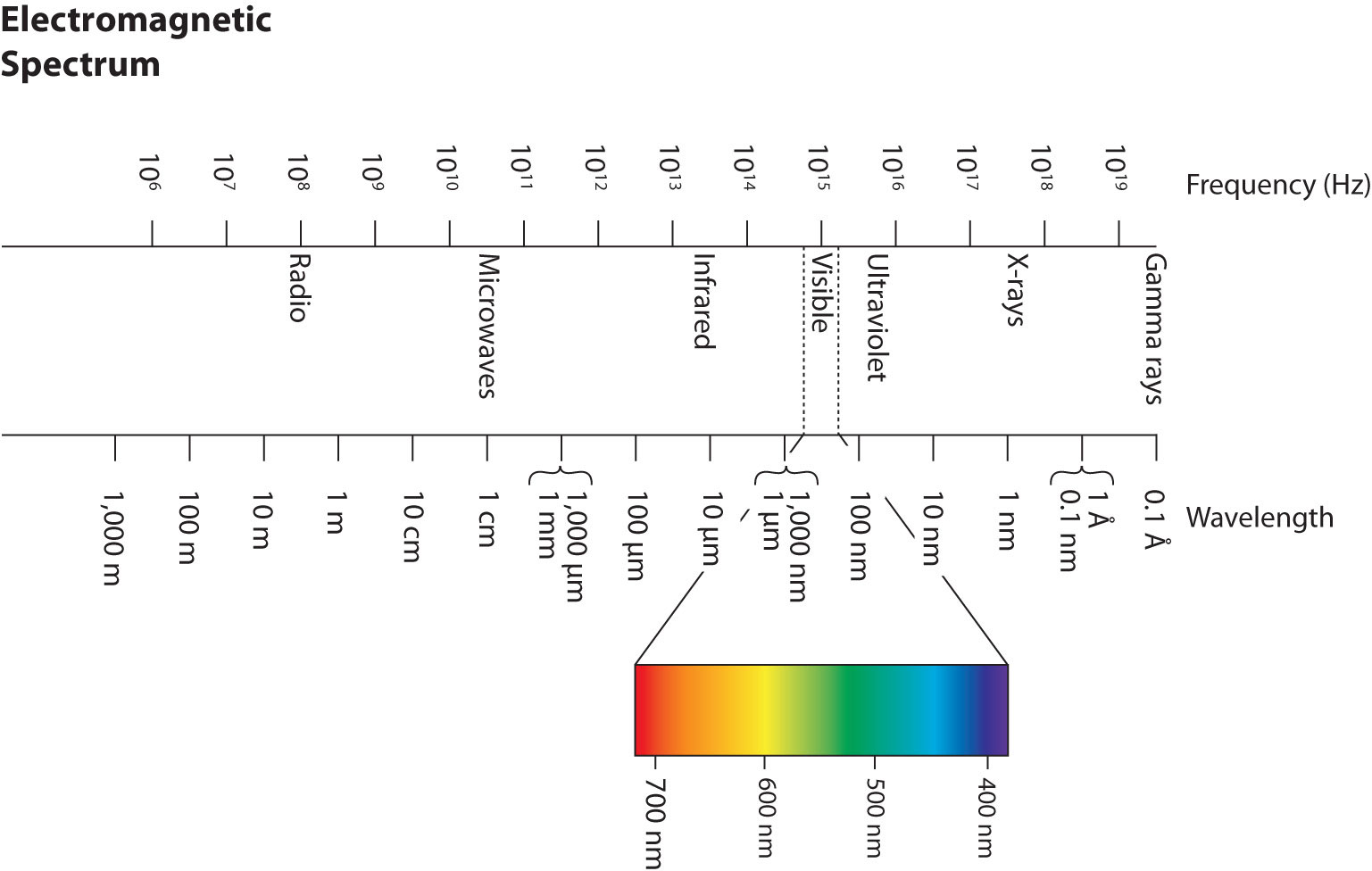
Being close to `10` `mu"m"`, I find that it's close to the infrared region. And if you convert to `"cm"^(-1)`, you should get `"1070.32 cm"^(-1)`, which is within the "fingerprint" region of the IR spectrum.