Calculate the wavelength of the first line in lyman series of the hydrogen spectrum (R = 109677 cm-1) how to do this?
2 Answers
Explanation:
where,
R = Rydbergs constant (Also written is
Z = atomic number
Since the question is asking for
since the electron is de-exited from
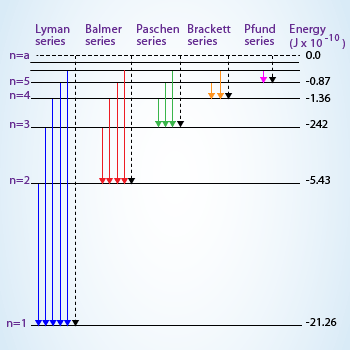
Therefore plugging in the values
Since the atomic number of Hydrogen is 1.
By doing the math, we get the wavelength as
since
therefore
or
Explanation:
The first emission line in the Lyman series corresponds to the electron dropping from
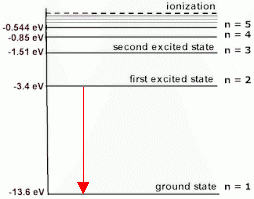
(Adapted from Tes)
The wavelength is given by the Rydberg formula
#color(blue)(bar(ul(|color(white)(a/a) 1/λ = -R(1/n_f^2 -1/n_i^2)color(white)(a/a)|)))" "#
where
For a positive wavelength, we set the initial as
#= "109 677" × 10^7color(white)(l) "m"^"-1" (1/4-1/1)#
#= "109 677 cm"^"-1" × (1-4)/(4×1)#
#= -"109 677 cm"^"-1" × (-3/4) = "82 257.8 cm"^"-1"#
#= "1.215 69" × 10^"-7"color(white)(l) "m"#
#= ul"121.569 nm"#