Question

How can we convert `DeltaH_f^@` at 298K to `DeltaH_f^@` at different temperatures ?? Also tell how to convert bond energy at 298K to bond energy at different temperature

Answer 1

You'd need to heat the product from standard temperature to nonstandard temperature at the same pressure and add that heating enthalpy.

However, I don't think bond enthalpy changes significantly at a different temperature. We can think of it like an activation energy for that particular molecule's dissociation reaction (in the gas phase to keep it simple):

`"AB"(g) -> "A"(g) + "B"(g)`

Activation energy is almost completely temperature independent, so we can assume that so is bond enthalpy.

---

Suppose you have the `DeltaH_f^@` for `"NH"_3` at `"298.15 K"`, for the reaction

`3"H"_2(g) + "N"_2(g) -> 2"NH"_3(g)`,

and suppose the reactant concentrations were constantly replenished such that the equilibrium is constantly pushed way towards `"NH"_3`.

The `DeltaH_f^@` (`=DeltaH_"rxn"^@` since all reactants were in their standard states) for `"NH"_3(g)` in this reaction is `-45.94 pm 0.35` `"kJ/mol"` from the NIST webbook.

This is the thermodynamic cycle for this process, to get the big picture:

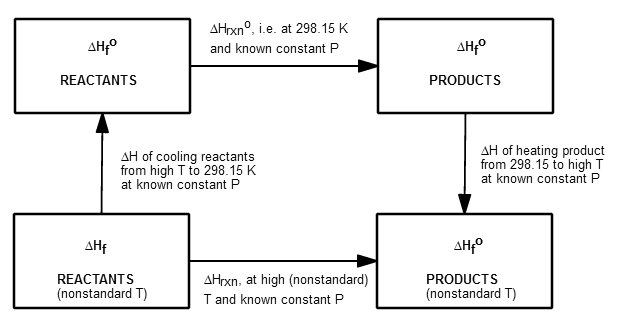
Usually we know `DeltaH_"rxn"^@`. To fill in `DeltaH` for heating curves, we have the following formula for heating from `T_1` to `T_2`:

`DeltaH_(T_1)^(T_2) = int_(T_1)^(T_2) C_P dT`

This requires that you know the constant-pressure heat capacity of
your compound(s) and that you assume that it stays constant in the relevant temperature range.

`C_P` can be looked up via the NIST webbook. For example, `"NH"_3` has:

http://webbook.nist.gov/cgi/cbook.cgi?ID=C7664417&Type=JANAFG&Plot=on

a `C_P` of `~~ "35.64 J/mol"cdot "K"` at `"298.15 K"` and `~~ "42.01 J/mol"cdot"K"` at `"500 K"`.

So, from using the average `C_P` in between the two temperatures, heating `"NH"_3` would approximately contribute a `DeltaH` of:

`DeltaH_(298.15)^(500) = int_(298.15)^(500) (42.01 + 35.64)/2 dT`

`~~ 38.825T_2 - 38.825T_1`

`= 38.825DeltaT`

`=` `38.825(500 - 298.15)`

`=` `"7836.83 J/mol"` `->` `"7.937 kJ/mol"`

(notice the resemblance to "`q = mc_sDeltaT`".)

The `DeltaH_f^@` for `"NH"_3(g)` was `-45.94 pm 0.35` `"kJ/mol"` from the NIST webbook. So, at `"500 K"`, it would be approximately:

`(-45.94 + 7.937)` `"kJ/mol"` `~~ color(blue)(-"38.10 kJ/mol")`

It's less negative at a higher temperature, meaning that it is less exothermic to form `"NH"_3(g)` at higher temperatures.

This makes physical sense because we are just assuming the heat released due to making it at a lower temperature is re-absorbed to heat the surroundings further (thus making the enthalpy released less negative).