Question

How do you express `cos theta - cos^2 theta + cot theta` in terms of `sin theta`?

Answer 1

`color(blue)(=>(sqrt(1-sin^2 theta) / sin theta) * ( sin theta - sin(2theta) / 2 - 1)`

Explanation:

`cos theta - cos^@ theta - cot theta`


`=>cos theta - cos^2 theta - (cos theta / sin theta)`

`=>cos theta * ( 1 - cos theta - (1/sin theta))`

`=>cos theta (sin theta - sin theta cos theta - 1 ) / sin theta`

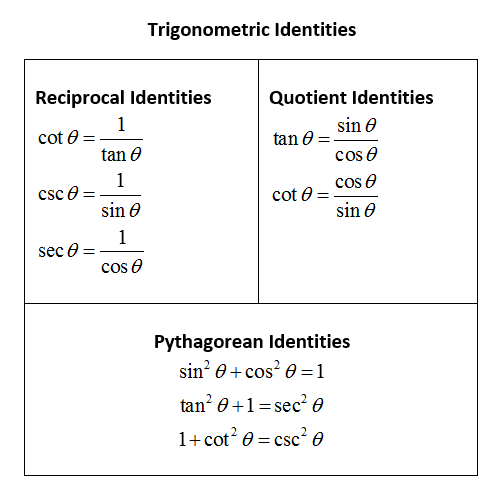
We will use the following identities to convert into sine form.

`color(crimson)(sin 2 theta = 2 sin theta cos theta, cos^2 theta = 1 - sin^2 theta`

`color(blue)(=>(sqrt(1-sin^2 theta) / sin theta) * ( sin theta - sin(2theta) / 2 - 1)`