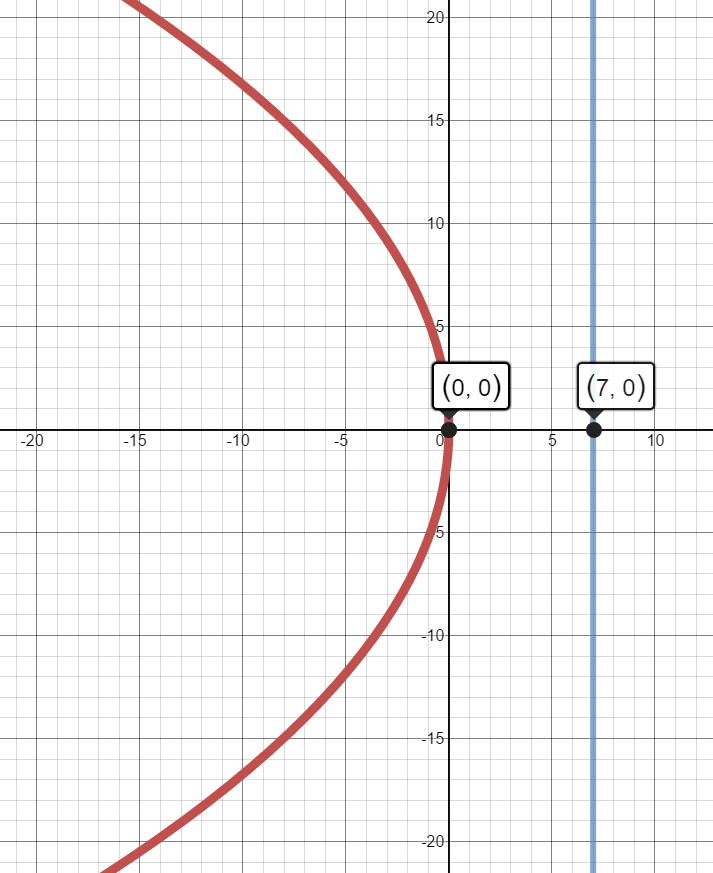
How do you find the equation of the parabola vertex at the origin and the directrix at x=7?
1 Answer
Jan 10, 2016
With a vertex
Explanation:
The absolute distance between the directrix and vertex
So, the coefficient
The sign of the coefficient must be NEGATIVE because the parabola opens to the left.
vertex
Finally, substitute the values into the equation ...
Equation :
hope that helped