Standard Form of the Equation
Key Questions
-
I believe you mean standard form. Standard form has another name, vertex form.
This is a duplicate question, please read the link below as it also has an example: How do I convert the equation
#f(x)=x^2+6x+5# to vertex form? -
The directrix of the parabola is a straight line that, together with the focus (a point), is used in one of the most common definition of parabolas.
In fact, a parabola can be defined as *the locus of points#P# such that the distance to the focus#F# equals the distance to the directrix#d# .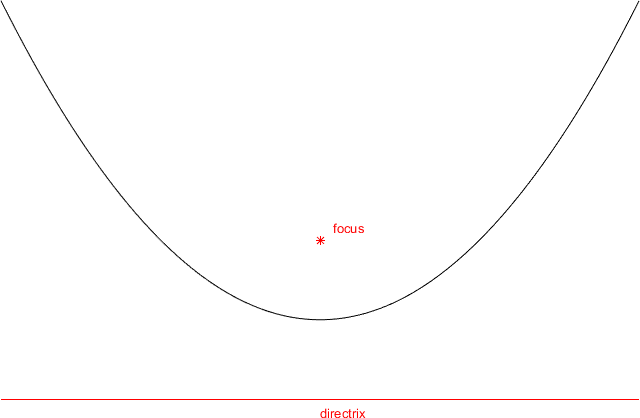
The directrix has the property of being always perpendicular to the axis of symmetry of the parabola.
-
Answer:
See the explanation section.
Explanation:
The term "standard form" is perhaps overused in mathematics.
The standard form for a quadratics function (as a polynomial function) is
#f(x)=ax^2+bx+c# .The standard for for the equation of a parabola (also called the vertex form) is like the standard form for other conic sections.
For a parabola with vertex
#(h,k)# through the points#(h+-1,k+a)# ,
(that is, it opens up or down)
the standard form is#y = a(x-h)^2 + k# (If the parabola opens sideways, it includes the points
#(h+a, k+-1)# and has form#x = a(y-k)^2+h# .)