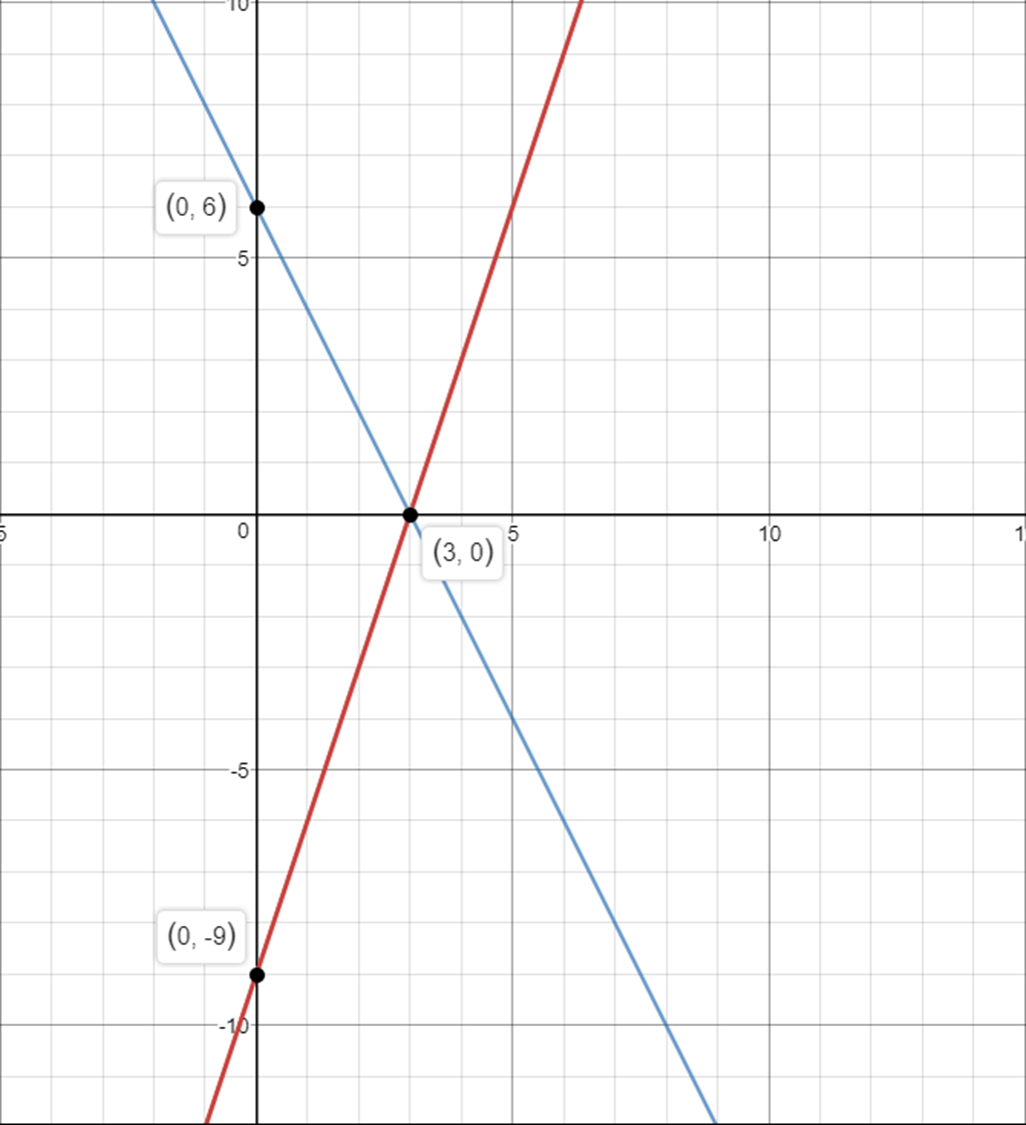
How do you solve the system of equations by graphing and then classify the system as consistent or inconsistent #3x-y=9# and #2x+y=6#?
1 Answer
Jan 8, 2018
#(3,0)#
Explanation:
Given -
#3x-y=9# -------------(1)
#2x+y=6# -------------(2)
If the slopes are different, both are consistent, else inconsistent.
When the equations are in the form
#ax+by =c#
The formula for slope is#m=- a/b#
Slope of the first line#m_1=- 3/(-1)=3#
Slope of the second line#m_2=- 2/1=2#
The slopes are different. They are consistent.
We have to find the intercepts for the two lines to graph them
y-intercept of the 1st line
#3(0)-y=9#
#y=-9#
#(0, -9)#
x-intercept of the 1st line
#3x-(0)=9#
#x=9/3=3#
#(3,0)#
y-intercept of the 2nd line
#2(0)+y=6#
#y=6#
#(0,6)#
x-intercept of the 2nd line
#2x+0=6#
#x=6/2=3#
#(3, 0)#
[