Question

How do you solve the system `y = - 2/3x` and `2x + 3y = 5` by graphing?

Answer 1

There is no solution to this system of equations

Explanation:

You have two equations. What is normally meant by 'solve' is really the question: What are the value for `x` and `y` where the two plots cross (coincide). This means that there are particular values for `x" and "y` that are true for both equation. Ok, lets look at the graph!

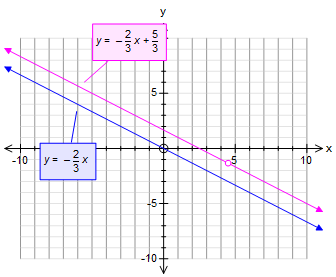
So the question now is: Why do they not cross?

Consider`" "2x+3y=5`

This can be re-written as `y=-2/3 x+5/3`

Now compare this to `" " y=-2/3 x`

Notice that the coefficient in front of `x` in both equations is the same. That is `-2/3`

This is the gradient. As the gradient (slope) is the same for both equations they are parallel.

This means that they NEVER cross. So they do NOT share the same values at any point.

`color(magenta)("Thus there is NO SOLUTION!")`