How do you solve # y=-x-10#, #9x-8y=-56# by graphing and classify the system?
1 Answer
Plot the graphs for both equations, then note if the corresponding lines intersect, are parallel, or co-linear.
(In this case they intersect and so the equations are consistent).
Explanation:
Find A Pair of Sample Solution Points for Each Equation
#{: (,color(red)(y=-x-10),,color(white)("XXXXXXXXXX"),,color(blue)(9x-8y=-56),), (color(white)("X")color(red)(x),,color(white)("X")color(red)(y),,color(white)("X")color(blue)(x),,color(white)("X")color(blue)(y)), (color(white)("X")color(red)(0),,color(red)(-10),,color(white)("X")color(blue)(0),,color(white)("X")color(blue)(7)), (color(red)(-10),,color(white)("X")color(red)(0),,color(blue)(-8),,color(blue)(-2)) :}#
Plot and Draw the Lines for Each Equation
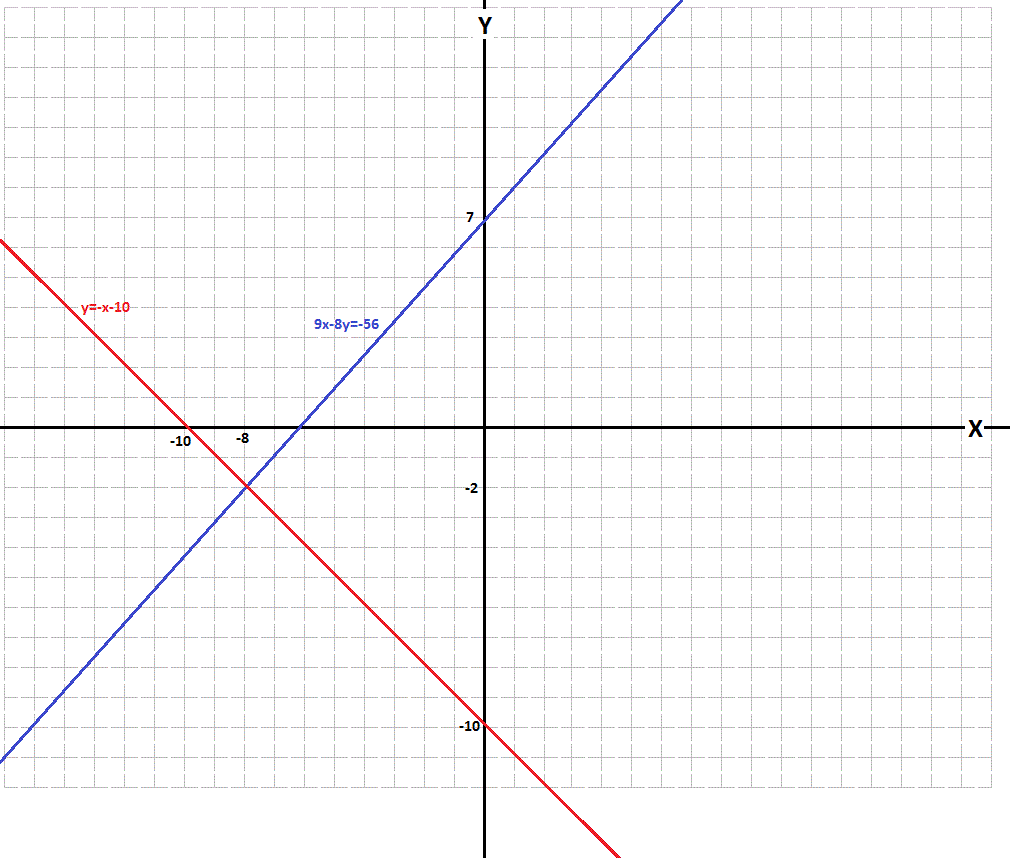
Note That the Lines Intersect
Since the lines intersect [at

