How do you tell whether the graph opens up or down, find the vertex, and find the axis of symmetry given y=1/2x^2+x-2?
1 Answer
The graph (the Parabola) Opens Up, as the coefficient of
Vertex 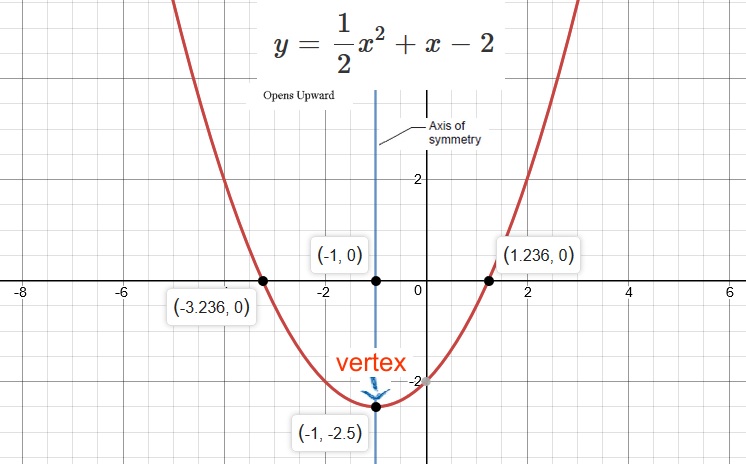
Explanation:
Standard Form of a Quadratic Equation is given by
If
Since in our Quadratic Equation, the coefficient of the
Also observe that, if the Parabola "Opens Upward" then our Vertex will be a "Minimum" of the Quadratic Function.
We are given the Quadratic Function
The expression
To obtain the y-coordinate value of the "Vertex", substitute the value obtained from using the expression
Hence,
After simplification, we get
Hence our Vertex
And our Axis of Symmetry
Please refer to the attached graph for a visual proof of our solution.
I hope this explanation is helpful.

