Consider vertex form, #y = a(x - p)^2 + q#. The vertex is at #(p, q)#. Now consider the graph of #y = x^2#, as shown directly below.
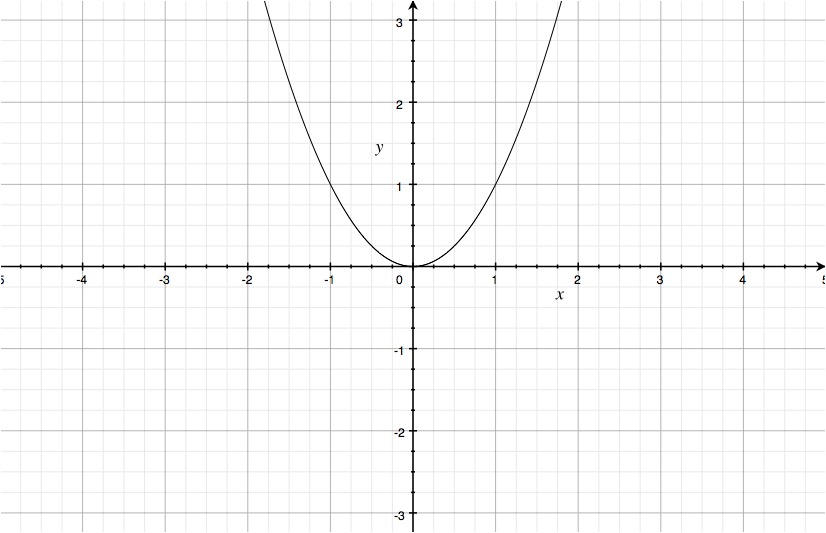
The vertex is at the origin, or in other words at #(0, 0)#.
Since this graph is neither stretched nor compressed, the parameter a is #1#. Hence, the equation of this graph, in non simplified form is #y = 1(x - 0)^2 + 0#.
Knowing that parameter #a# controls shape, and knowing that our new function has the same shape as #y = x^2#, we also know that #a = 1# in our new equation. The vertex is at #(-3, 2)#, so replacing the #(0, 0)# inside the equation #y = 1(x - 0)^2 + 0#, we have #y = 1(x - (-3))^2 + 2#, or, better simplified, #y = (x + 3)^2 + 2#.
Hence, the equation of the parabola with the same shape as #y = x^2# and a vertex at #(-3, 2)# is #y = (x + 3)^2 + 2#.
Hopefully this helps!


