Question

How to solve the system of equations `2x-y=-1` and `3x+2y=-5` by graphing?

Answer 1

From the graphs, we get point of intersection at 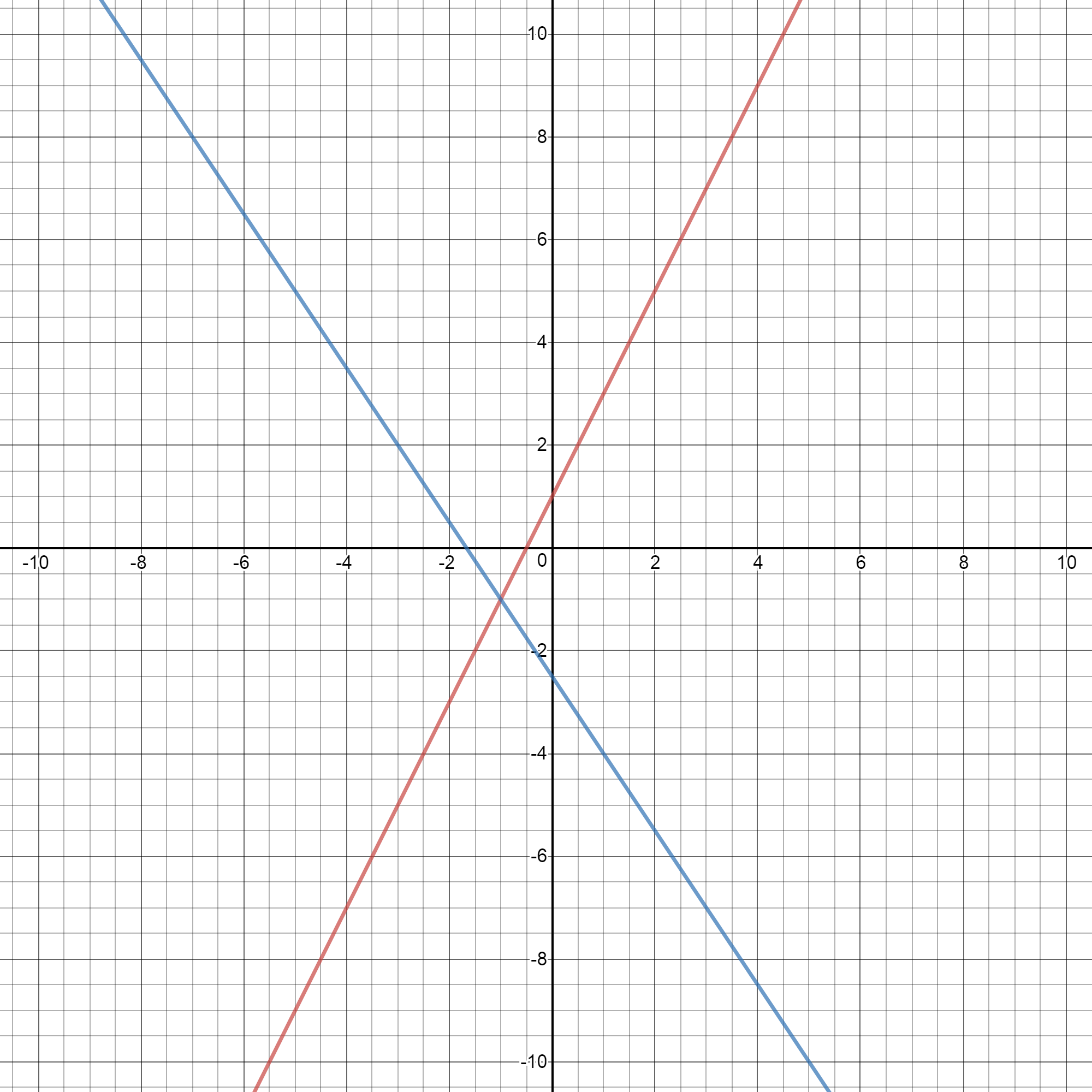`x=-1` and `y=-1`.

Explanation:

The intercept of two linear graphs is the solution for `x` and `y`.

Lets take the first equation:
`2x-y=-1`

Find the `x` intercept and `y` intercept and plot the graph.

`x`-intercept, `y=0`
`2x-y=-1`

`2x-0=-1`

`2x=-1`

`x=-1/2` ------> `x`-Intercept

`y`-intercept, `x=0`

`2x-y=-1`

`2(0)-y=-1`

`-y=-1`

`y=1`------>`y`-Intercept

Lets take the second equation:
`3x+2y=-5`

Find the `x` intercept and `y` intercept and plot the graph.

`x`-intercept, `y=0`
`3x+2y=-5`

`3x+2(0)=-5`

`3x=-5`

`x=-5/3` -----> `x`-Intercept

`y`-intercept, `x=0`
`3x+2y=-5`

`3(0)+2y=-5`

`2y=-5`

`y=-5/2`------> `y`-Intercept

**Now plot the two linear graphs and from the graph, the intersection of these tow linear graphs are the value of `x` and `y` satisfying both the equations. Graphs are shown below:

From the graphs, we get point of intersection at `x=-1` and `y=-1`.