Question

What are all the possible rational zeros for `f(x)=x^3+x^2-5x+3` and how do you find all zeros?

Answer 1

1,1, -3

Explanation:

Substituting x=1 makes f(x)= 1+1-5+3 =0 hence x=1 is one of the rational zeros.

Since x=1 is a zero, x-1 would be a factor of f(x). therefore divide f(x) by x-1 by using long or synthetic division.

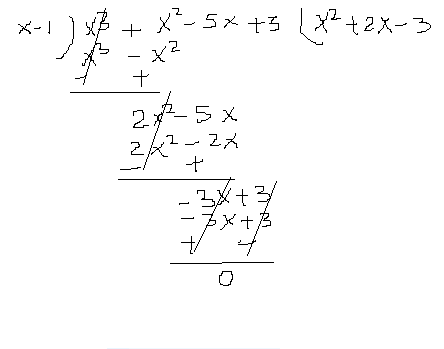
So, `f(x)= (x-1)(x^2+2x-3)`

Now for finding other zeros of f(x), put `x^2+2x-3=0` and solve for x.

This quadratic equation can be solved by factorisation or using quadratic formula.

Factorisation is quite easy. Writing 2x as 3x-x, the quadratic expression becomes `x^2 +3x -x-3=0`

`x(x+3) -1(x+3)=0`

(x-1)(x+3)=0 giving x= 1, -3

Thus all the zeros of f(x) are now known. These are, 1( which is a repeated zero) and -3