What are the vertex, focus and directrix of # 9y=x^2-2x+9 #?

Question

1420 views around the world

You can reuse this answer
Creative Commons License

Answer 1 · 2017-11-21T07:23:52

Vertex #(1, 8/9)#
Focus #(1,113/36)#
Directrix #y=-49/36#

Given -

#9y=x^2-2x+9#

vertex?
Focus ?
Directrix?

#x^2-2x+9=9y#

To find Vertex, Focus and directrix, we have to rewrite the given equation in vertex form i.e., #(x-h)^2=4a(y-k)#

#x^2-2x=9y-9#

#x^2-2x+1=9y-9+1#

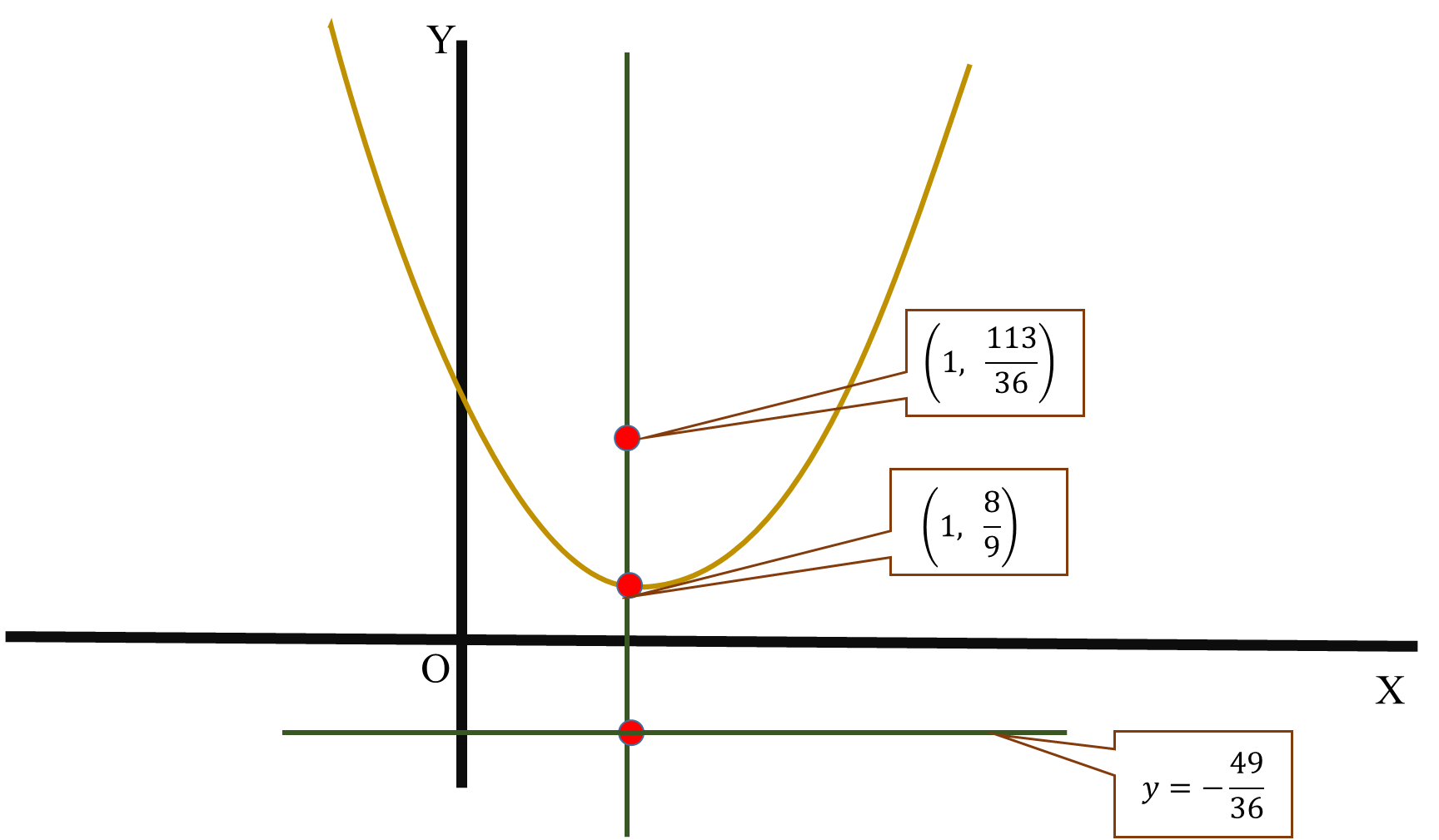
#(x-1)^2=9y-8#

#(x-1)^2=9(y-8/9)#

==================
To find the equation in terms of #y# [This not asked in the problem]

#9(y-8/9)=(x-1)^2#

#y-8/9=1/9.(x-1)^2#

#y=1/9.(x-1)^2+8/9#

================

Let us uses #9(y-8/9)=(x-1)^2# to find the vertex, focus and directrix.

#(x-1)^2=4 xx 9/4(y-8/9)#

Vertex #(1, 8/9)#

Focus #(1,(8/9+9/4))#
Focus #(1,113/36)#
Directrix #y=8/9-9/4#
Directrix #y=-49/36#

enter image source here