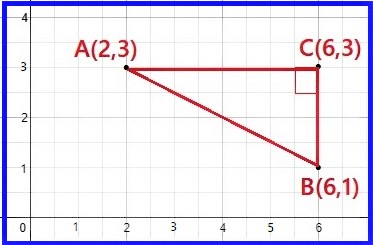
What is the orthocenter of a triangle with corners at #(2 ,3 )#, #(6 ,1 )#, and (6 ,3 )#?
1 Answer
Jun 16, 2018
Hence, the orthocentre of
Explanation:
Let ,
We take,
So,
It is clear that,
Hence,
Hence, the orthocentre of
Please see the graph: