What is the orthocenter of a triangle with corners at #(9 ,7 )#, #(6 ,9 )#, and (2 ,4 )#?
1 Answer
Orthocenter is at
Explanation:
For convenience label the points on this triangle as in the diagram below:
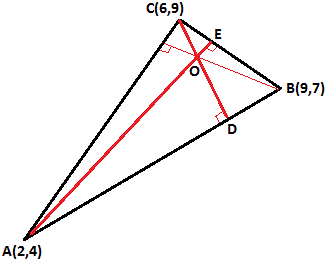
Note that (by definition) the orthocenter of a triangle is the point where the altitudes meet (labelled
Slope(
Slope(
Equation of line (
Slope(
Slope(
Equation of line (
Solving the system of equations:
and
