Question

What is the speed of a visible light wave if the wavelength is `4 * 10^-7` `m` and frequency `7.5 * 10^14` `Hz`?

Answer 1

`3 * 10^8"m s"^(-1)`

Explanation:

As you know, frequency and wavelength have an inverse relationship described by the equation

`color(blue)(lamda * nu = c)" "`, where

`lamda` - the wavelength of the light wave
`nu` - its frequency
`c` - the speed of light

Now, in a vacuum, the speed of light is usually given as `3 * 10^8"m s"^(-1)`. If your light wave is traveling in a different medium, you can expect variations from this values.

Keep in mind that all these variations will result in values that are smaller than that recorded for the speed of light in a vacuum.

The inverse relationship that exists between frequency and wavelength can be deduced from the values given to you. Notice that a relatively high frequency corresponds to a relatively short wavelength,

![)

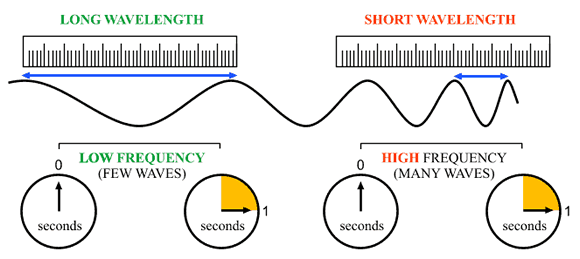
So, if you multiply the values given to you and end up with a value for the speed of light that is smaller than `3 * 10^8 "m s"^(-1)`, you can conclude that the light wave is not traveling in a vacuum.

If you wend up with a value that is bigger than `3 * 10^8"m s"^(-)`, you can conclude that the values given to you are incorrect.

One more thing before doing the calculation - notice that the frequency is given to you in Hertz, `"Hz"`. As you know,

`"1 Hz" = 1/"s" = "1 s"^(-1)`

Since the wavelength of the wave is given in meters, you're going to have to use this unit for frequency in order to get `"m s"^(-1)` for the speed.

So, plug in your values to get

`c = 4 * 10^(-7)"m" * 7.5 * 10^(14)"s"^(-1) = 30 * 10^(7)"m s"^(-1)`

This is of course equivalent to

`c = color(green)(3 * 10^8"m s"^(-1))`

Your light wave is traveling in a vacuum.