What is the vertex form of the equation of the parabola with a focus at (1,20) and a directrix of #y=23 #?

Question

1897 views around the world

You can reuse this answer
Creative Commons License

Answer 1 · 2017-10-04T05:54:39

#y=x^2/-6+x/3+64/3#

Given -

Focus #(1,20)#
directrix #y=23#

The vertex of the parabola is in the first quadrant. Its directrix is above the vertex. Hence the parabola opens downward.

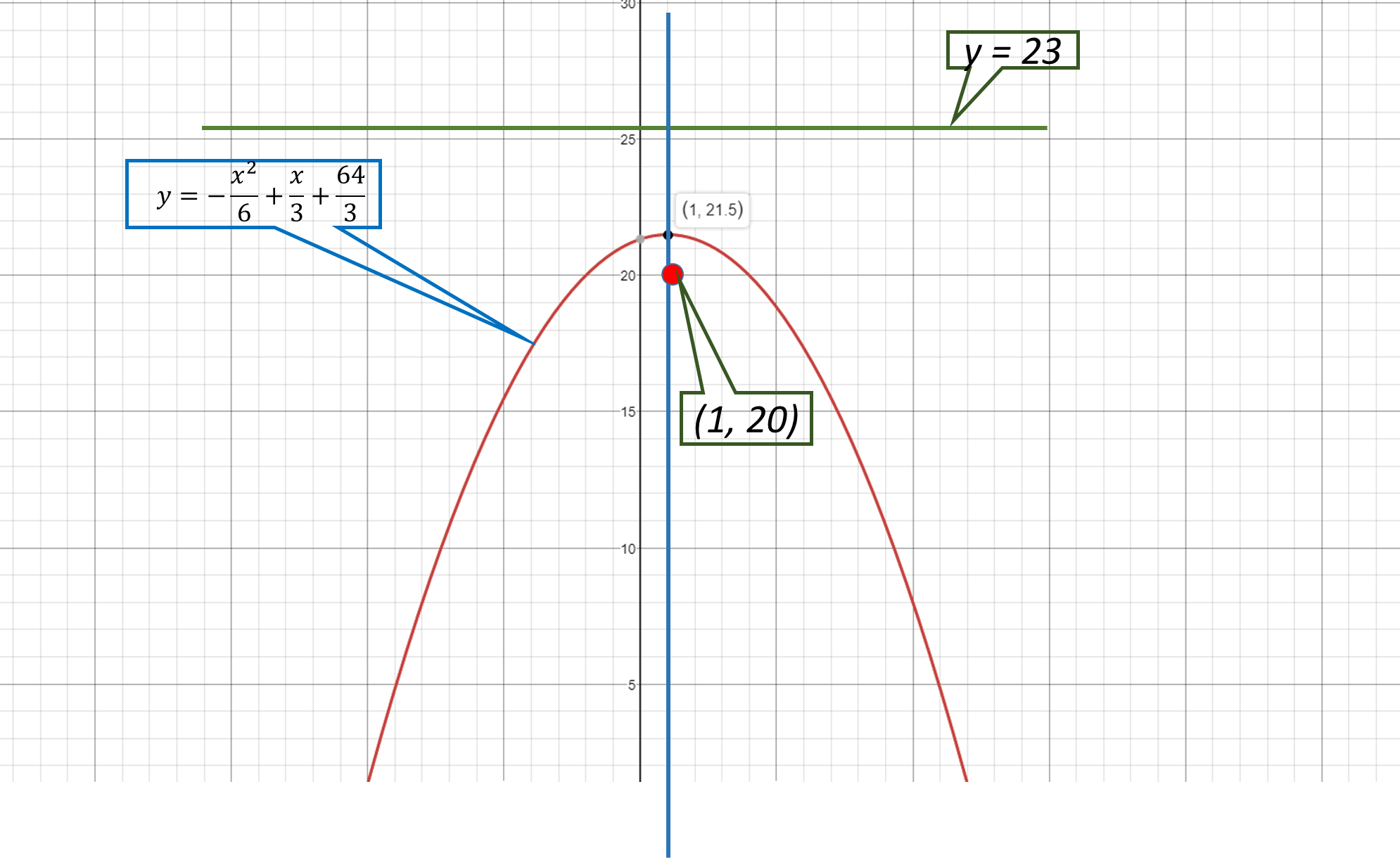
enter image source here

The general form of the equation is -

#(x-h)^2=--4xxaxx(y-k)#

Where -

#h=1# [ X-coordinate of the vertex]
#k=21.5# [ Y-coordinate of the vertex]

Then -

#(x-1)^2=-4xx1.5xx(y-21.5)#

#x^2-2x+1=-6y+129#
#-6y+129=x^2-2x+1#
#-6y=x^2-2x+1-129#
#y=x^2/-6+x/3+128/6#
#y=x^2/-6+x/3+64/3#