What is the y value of the intersection of #x + y = 8# and #x – 2y = -4# when solving by using the graphing method?

Question

7005 views around the world

You can reuse this answer
Creative Commons License

Answer 1 · 2018-03-22T09:49:34

#y=4#

First rearrange the two equations so #y# is a function of #x#:

#x+y=8-> \ \ \ \ \ \ \ \color(blue)(y=8-x) \ \ \ \ \ \ \ \ [ 1 ]#

#x-2y=-4->color(blue)(y=1/2x+2) \ \ \ \ [ 2 ]#

Because these are straight lines, we only need to put in two values of #x# for each equation and then calculate the corresponding values of #y#.

#[1] \ \ \ \ # #x=-2 \ \ \ , x=6#

#y=8-(-2)=10#

#y=8-(6)=2#

So we have coordinates #(-2,10)# and #(6,2)#

#[2] \ \ \ \ # #=-4 \ \ \ # , #x=6#

#y=1/2(-4)+2=0#

#y=1/2(6)+2=5#

So we have coordinates #(-4,0)# and #(6,5)#

We now plot each pair of coordinates and join them with a straight line.

You should have a graph that looks like this:
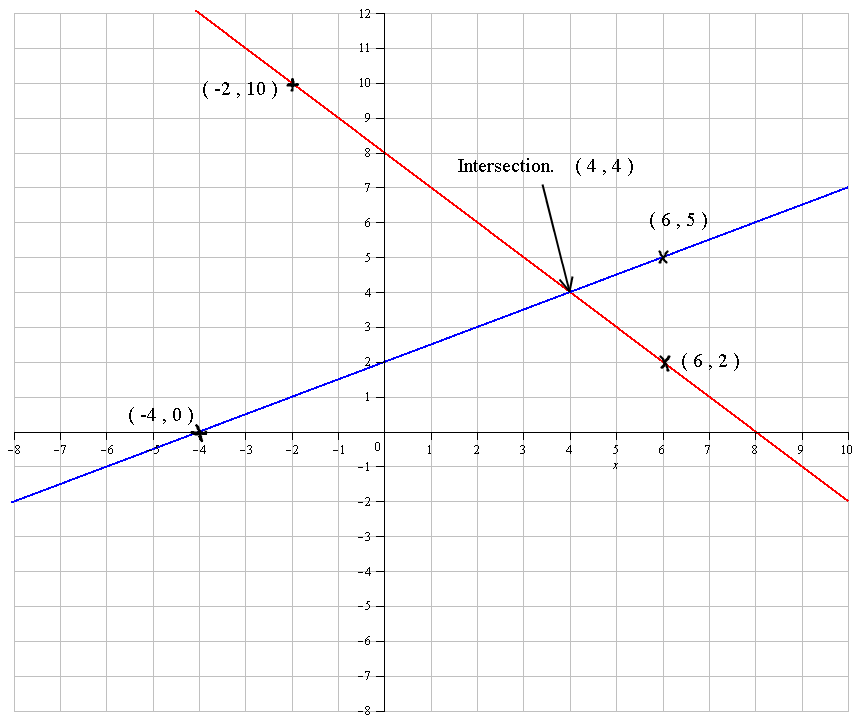
enter image source here

We can see from this that, at the intersection the #y=4#