How do you graph and solve # 3|x-1|+2>=8#?

Question

2149 views around the world

You can reuse this answer
Creative Commons License

Answer 1 · 2015-12-15T07:41:12

#(-oo, -1] " U " [ 3, oo) #

#|x| >= k => " " x>= k " " or " " " x<= -k#

#|x| <= k => " " k <=x <= k#

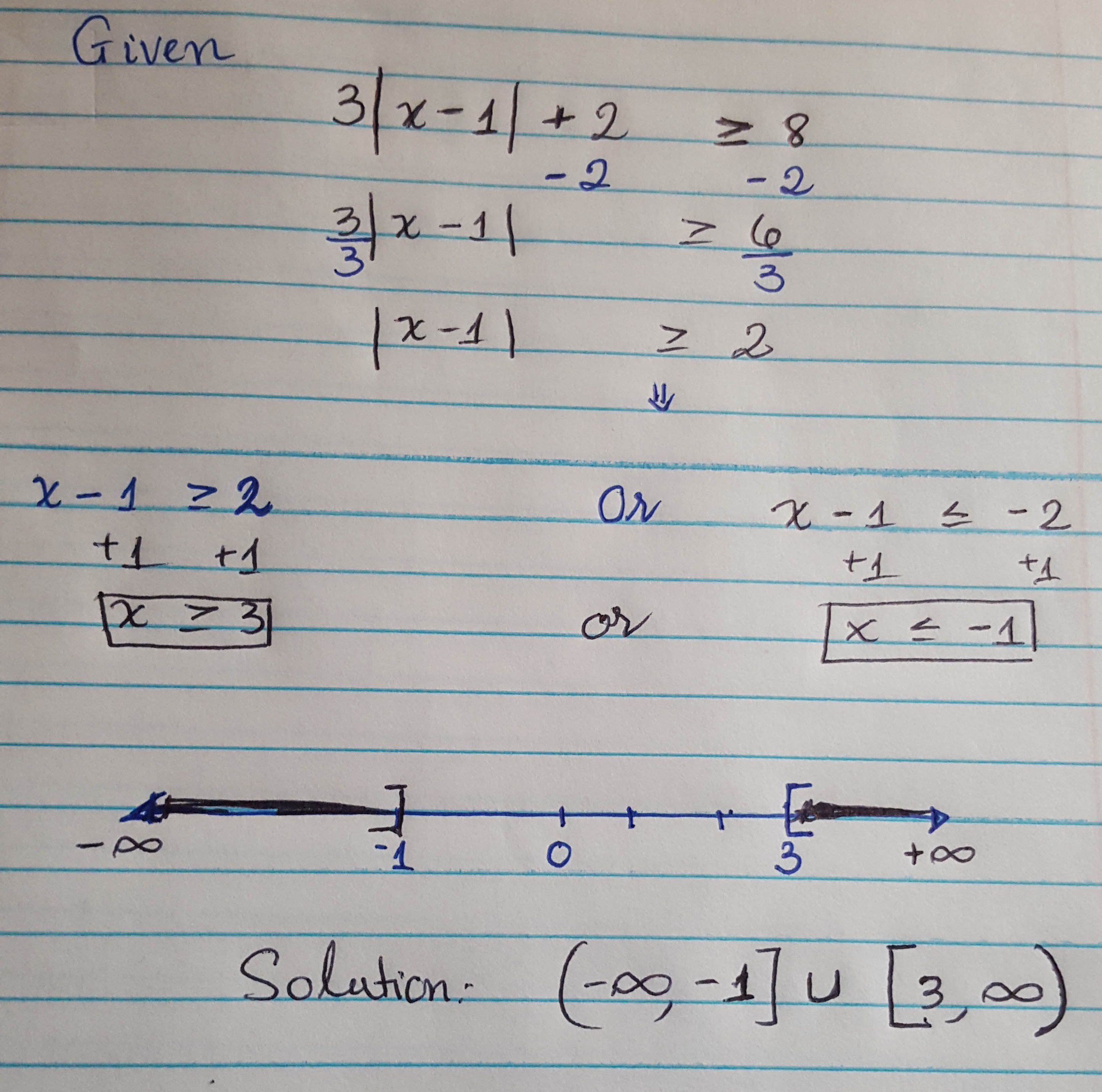
Given:

#3|x-1| +2 >= 8#

Isolate the inequality, so absolute value can be by itself.

Step 1: Subtract 2 to both side

#3|x-1|+cancel(2 color(red)(- 2)) >= 8 color(red)(- 2)#

#3|x-1| >= 6#

Step 2 : Divide by 3 to both side

#(3|x-1|)/color(blue)(3) >= 6/color(blue)(3)#

#|x-1| >= 2#

Step 3 : Undo the absolute value like the information mention at the beginning

#=>x-1 >= 2 " " " " or " " " " x-1 <= -2#

Step 4 : Solve for #x#

# x >= 3 " " " or " " " x<= -1#

Step 5: Draw the number line and determine the interval from Step 4.

Solution: #(-oo, -1] " U " [ 3, oo) #
enter image source here