What is the area of a trapezoid whose diagonals are each 30 and whose height is 18?

Question

What is the area of a trapezoid whose diagonals are each 30 and whose height is 18?

1 Answer

Impact of this question

7862 views around the world

You can reuse this answer
Creative Commons License

Answer 1 · 2016-01-05T16:01:36

$S_{t r a p e z o i d} = 432$ $S_(trapezoid)=432$

Explanation:

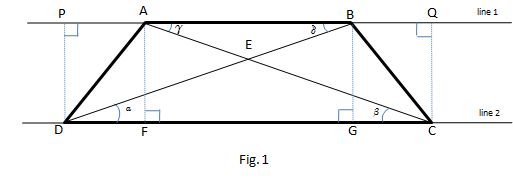
Consider Figure 1

![I have created this figure using MS Excel]
( useruploads.socratic.org )

In a trapezoid ABCD that satisfies the conditions of the problem (where $B D = A C = 30$ $BD=AC=30$ , $D P = 18$ $DP=18$ , and AB is parallel to CD) we notice, applying the Alternate Interior Angles Theorem, that $α = δ$ $alpha=delta$ and $β = γ$ $beta=gamma$ .

If we draw two lines perpendicular to segment AB, forming segments AF and BG, we can see that $△_{A F C} \equiv △_{B D G}$ $triangle_(AFC)-=triangle_(BDG)$ (because both triangles are right ones and we know that the hypotenuse of one is equal to the hypotenuse of the other and that a leg of one triangle is equal to a leg of the other triangle) then $α = β$ $alpha=beta$ => $γ = δ$ $gamma=delta$ .

Since $γ = δ$ $gamma=delta$ we can see that $△_{A B D} \equiv △_{A B C}$ $triangle_(ABD)-=triangle_(ABC)$ and $A D = B C$ $AD=BC$ , therefore the trapezoid is isosceles.
We also can see that $△_{A D P} \equiv △_{B C Q}$ $triangle_(ADP)-=triangle_(BCQ)$ => $A P = B Q$ $AP=BQ$ (or $x = y$ $x=y$ in figure 2).

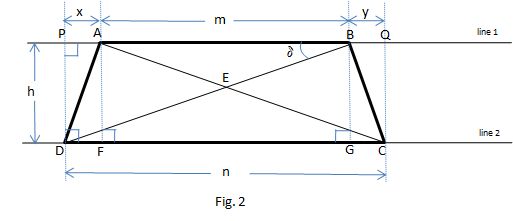
Consider Figure 2

![I have created this figure using MS Excel]
( useruploads.socratic.org )

We can see that the trapezoid in figure 2 has a different shape than the one in figure 1, but both satisfy the conditions of the problem. I presented this two figures to show that the information of the problem doesn't allow to determine the sizes of the base 1 ( $m$ $m$ ) and of the base 2 ( $n$ $n$ ) of the trapezoid, but we'll see that there's no need of more information to calculate the trapezoid's area.

In $△_{B D P}$ $triangle_(BDP)$
$D B^{2} = D P^{2} + B P^{2}$ $DB^2=DP^2+BP^2$ => $30^{2} = 18^{2} + {(x + m)}^{2}$ $30^2=18^2+(x+m)^2$ => ${(x + m)}^{2} = 900 - 324 = 576$ $(x+m)^2=900-324=576$ => $x + m = 24$ $x+m=24$

Since $n = m + x + y$ $n=m+x+y$ and $x = y$ $x=y$ => $n = m + 2 \cdot x$ $n=m+2*x$ and $m + n = m + m + 2 \cdot x = 2 \cdot (x + m) = 2 \cdot 24$ $m+n=m+m+2*x=2*(x+m)=2*24$ => $m + n = 48$ $m+n=48$

$S_{t r a p e z o i d} = \frac{b a s e_{1} + b a s e_{2}}{2} \cdot h e i g h t = \frac{m + n}{2} \cdot 18 = \frac{48 \cdot 18}{2} = 432$ $S_(trapezoid)=(base_1+base_2)/2*height=(m+n)/2*18=(48*18)/2=432$

Note: we could try to determinate m and n conjugating these two equations:
In $△_{A D P} \to A D^{2} = A P^{2} + h^{2}$ $triangle_(ADP) -> AD^2=AP^2+h^2$ => $A D^{2} = {(24 - m)}^{2} + 18^{2}$ $AD^2=(24-m)^2+18^2$
In $△_{A B D} \to A D^{2} = A B^{2} + B D^{2} - 2 \cdot A B \cdot B D \cdot cos δ$ $triangle_(ABD) -> AD^2=AB^2+BD^2-2*AB*BD*cos delta$ => $A D^{2} = m^{2} + 30^{2} - 2 \cdot m \cdot 30 \cdot (\frac{4}{5})$ $AD^2=m^2+30^2-2*m*30*(4/5)$
( $cos δ = \frac{4}{5}$ $cos delta =4/5$ because $sin δ = \frac{18}{30} = \frac{3}{5}$ $sin delta = 18/30=3/5$ )
But resolving this system of two equations, we would only discover that m and the side AD are indeterminate.

Science

Math

Humanities

... and beyond

What is the area of a trapezoid whose diagonals are each 30 and whose height is 18?

1 Answer

Explanation:

Related questions

Impact of this question